I'm pretty sure the expected answer to this problem involves an argument such
as the one suggested by @whuber.
However, because the values are specific, a grid search in R can be used to
find an exact solution, as shown below. I assume $3$ is the gamma rate parameter, as in R. [There is no general
agreement whether the second parameter of a gamma random variable is a 'rate' or 'scale' parameter, so you should always say which applies.]
n = 1:100 # hoping answer is < 101
p = pgamma(n/2, n, 3) # P(X_n < n/2) for X_n ~ Gamma(shape=n/2, rate=3)
min(n[p > .999]) # smallest n that meets condition
[1] 50
pgamma(50/2, 50, 3) # verification
[1] 0.9990961 # OK
pgamma(49/2, 49, 3)
[1] 0.9989981 # not OK
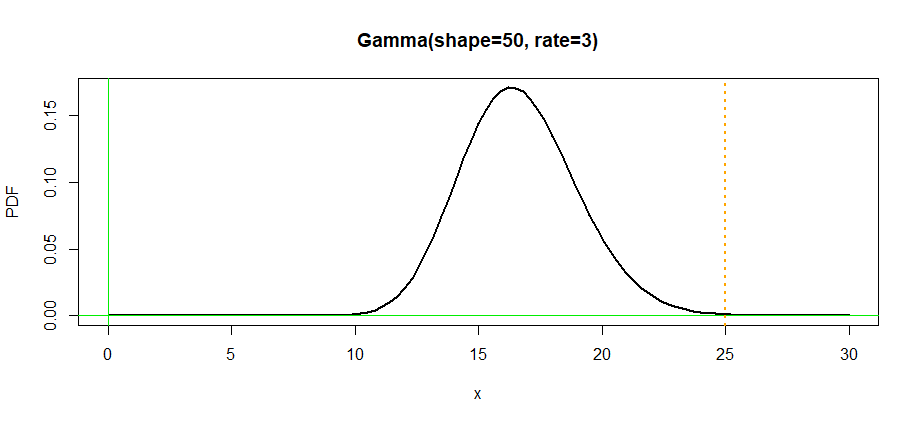
Graph:
curve(dgamma(x, 50, 3), 0, 30, lwd=2, ylab="PDF", main="Gamma(shape=50, rate=3)")
abline(h=0, col="green2")
abline(v=0, col="green2")
abline(v=25, col="orange", lwd=2, lty="dotted")
Note: If the scale is $3$ and rate is $1/3,$ then there is no positive integer $n$ that works:
pgamma(1/2, 1, 1/3)
[1] 0.1535183 # too small
pgamma(2/2, 2, 1/3)
[1] 0.04462492 # even smaller
pgamma(10/2, 10, 1/3)
[1] 1.011967e-05 # etc.

