Need help interpreting an answer about the Cauchy distribution and Huygens principle

Question

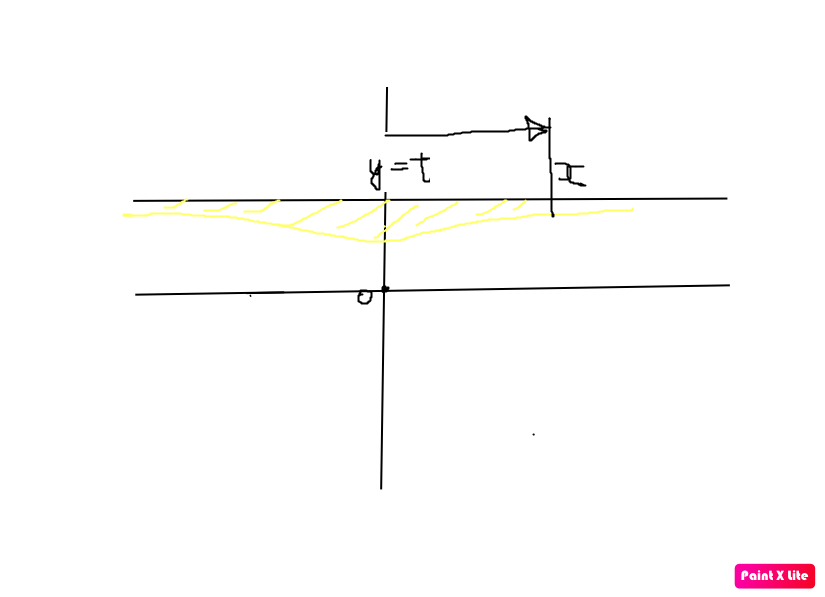
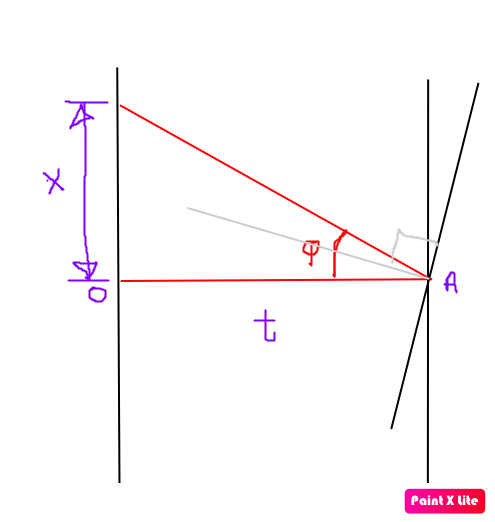
I'm trying to understand the answer here, which provides a physical interpretation for why the Cauchy distributions mean doesn't exist makes the following statement:

If a unit light source is located at the origin, then the distribution of the intensity of light along the line $y=t$.

The same statement is also found in the book, "An introduction to probability theory and its applications", volume 2 by Feller (footnote of page 51 in the first edition).

It seems to suggest that the intensity at a point $(x,t)$ is given by:

$$\gamma_t = \frac{t}{t^2+x^2}\frac{1}{\pi}\tag{1}$$

This isn't obvious to me. In the main text, Feller provides an example with a ray of light reflecting off a mirror that can be rotated and that follows a Cauchy distribution (described in detail here). But why is the intensity from a point source on a line given by equation (1) above?

Answer 1

The answer occurred to me once I posted this. Instead of deleting the question, I decided to leave it in and answer it for my future reference. Basically, from Fellers experiment described in the same page, we can think of point A as the point source of light (instead of the point that is reflecting it). Then, the conclusion naturally follows.