4
$\begingroup$

I want to do a study to examine if there is a correlation between nursing students' confidence & satisfaction, and their intuitiveness. I have two instruments, The Student Satisfaction and Self-Confidence in Learning questionnaire is a 13 item instrument which uses a five point scale to measure self confidence and satisfaction in nursing students and the Miller Intuitiveness questionnaire, which is an 18 item instrument which uses a six point scale. What statistical test can I use to see if there's a connection between the two?

Edit: I'm leaning towards Spearmans Rank Order correlation, can anyone confirm this is suitable please?

Improve this question
$\endgroup$
5
  • $\begingroup$ what software are you going to use to run this test? $\endgroup$
    – Eric Peterson
    Commented Apr 12, 2013 at 3:17
  • $\begingroup$ Is there a standard way of processing the results from each instrument into one or two dimensions eg a "self confidence index", "satisfaction index" and "intuitiveness index" - or are you going to look for connections at the level of individual questions? Also, I assume you will have a sample of subjects who completed both instruments? $\endgroup$
    – Peter Ellis
    Commented Apr 12, 2013 at 23:03
  • $\begingroup$ Yes, I'll have an index for all three, which I then want to compare and look for correlation. The idea is that everyone who fills it out will be a student nurse and will fill out both questionnaires, and then their three indexes will be compared, and I want to look for a correlation then between everyone's compared results. Does this make sense? I do not have a control group as the questionnaires are geared solely towards nurses, and thus would not be applicable for an alternative group. $\endgroup$
    – Rebeccapedia
    Commented Apr 12, 2013 at 23:18
  • $\begingroup$ I need your help on my statistics. I have a group of people with e types of diagnostics: 1. clinical and the 2nd hystopathological. Normally, these two diagnostics must be identical, but due to some clinical errors they aren't always. So whatI have to do is to find the corellation between the two, how many match and how many dont match. What test to I need to use? Thanks for you help, guys ! $\endgroup$
    – user42419
    Commented Mar 23, 2014 at 16:42
  • $\begingroup$ Welcome to the site, @Adelina. This isn't an answer to the OP's question. Please only use the "Your Answer" field to provide answers. If you have your own question, click the [ASK QUESTION] at the top & ask it there, then we can help you properly. Since you are new here, you may want to take our tour, which contains information for new users $\endgroup$
    – gung - Reinstate Monica
    Commented Mar 23, 2014 at 16:57

1 Answer 1

Reset to default
5
$\begingroup$

Yes. Spearman's correlation coefficient will probably be a good robust choice as it does not depend on a linear relationship between the variables. There are some nice illustrations in the Wikipedia article.

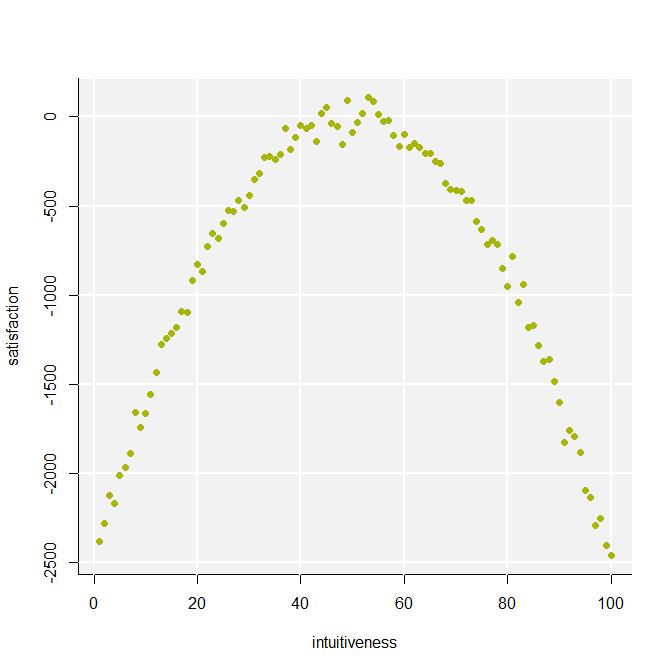
You should certainly draw pairwise scatterplots to show the three two-way relationships and alert you to any further problems that might suggest you need an alternative approach. For example, in the unlikely event the relationship is like that shown below, any correlation coefficient including Spearman's will be close to zero - a correlation simply can't cope with the situation where the direction of the relationship changes after a key point of maximum marginal return. If this is the case you will have no choice but to use a non-linear model.

enter image description here

When you want to test for significant evidence a correlation is different from zero, it is best to use a bootstrap. I don't know if SPSS can do this.

You don't need a control group, as you say.

Depending on how far you need to go into this, a more powerful alternative to a correlation coefficient is a model where one of the indexes is the response and the other two are explanatory variables, possibly allowing for an interaction between them. Your choice would depend on your research question.

Final suggestion - you might want to consider randomizing the order they fill in the questionnaires in case this has an impact.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thank you so much Peter, I really, really appreciate this! $\endgroup$
    – Rebeccapedia
    Commented Apr 13, 2013 at 1:08

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.