2
$\begingroup$

From another Cross Validated question

Given $X\sim N(\mu_X, \sigma_X^2)$ and $Y\sim N(\mu_Y, \sigma_Y^2)$ are independent, and you know $X+Y=s$. What is the expected value of $X$?

The question makes it sound like $Y=s-X$ yet $X$ and $Y$ are independent. How can this be?

$$cov(X,Y)\\=cov(X,s-X)\\=-var(X)\ne 0$$

Nonzero covariance is one form of dependence and precludes independence.

Improve this question
$\endgroup$
5
  • $\begingroup$ See if the image at stats.stackexchange.com/a/9073/2958 helps $\endgroup$
    – Henry
    Commented Aug 18, 2022 at 0:46
  • $\begingroup$ It isn't that the RVs are deterministically adding to $s$, rather the question is asking about conditioning on the event. $\endgroup$
    – Ben
    Commented Aug 18, 2022 at 0:46
  • $\begingroup$ @Ben That seems to be the approach taken in the other question, not an assumption of the problem faced in the interview. $\endgroup$
    – Dave
    Commented Aug 18, 2022 at 0:49
  • $\begingroup$ I agree with @Ben and have interpreted the questions as asking for the conditional expectation of $X$ given $X+Y=s$ for a specific (realized) $s$. $\endgroup$
    – statmerkur
    Commented Aug 18, 2022 at 0:56
  • $\begingroup$ Hi @Dave. Writing mathematical expressions in plain English necessarily leads to imprecision. "If $X+Y=s$, what is the expectation of $X$" is just slightly sloppy wording for a conditional expectation. $\endgroup$
    – Ben
    Commented Aug 18, 2022 at 1:56

2 Answers 2

Reset to default
6
$\begingroup$

Ben's comment pretty much says it all.

$X, Y$ being independent doesn't mean there cannot be an event where their added realisations have a particular aggregate, say $s.$ But the distinction must be noted: $$Y :\ne X-s;$$ the necessary condition of independence is uncorrelatedness, i.e. $\mathbb{Cov}(X, Y) = 0,$ but in no way $Y$ is defined to be $X- s. $

Improve this answer
$\endgroup$
2
$\begingroup$

This sounds a bit like the idea behind collider bias, the reverse of confounding bias.

  • Confounding bias: x and y are found to be correlated because both are caused by z.
  • Collider bias: x and y are found to be correlated because they both cause z and there is a selection effect based on z.

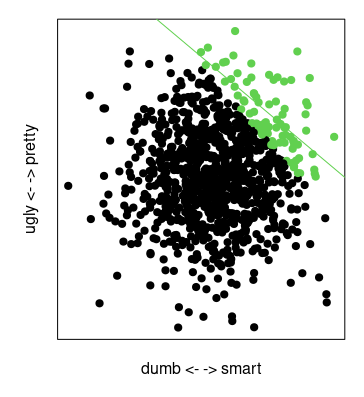
example of bias

Image from the question:Can spurious correlations exist in the (theoretical) population?

So $X$ and $Y$ may not be correlated, but when you condition on $s$ then they are correlated.

It is written a bit implicitly in the interview question but the statement implies a conditioning

Given that ... and you know $X+Y=s$

You might assume that this means a particular sampled individual case has $X+Y=s$. Otherwise there is a contradiction. $X$ and $Y$ can not be independent while also $X+Y=s$.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.