0
$\begingroup$

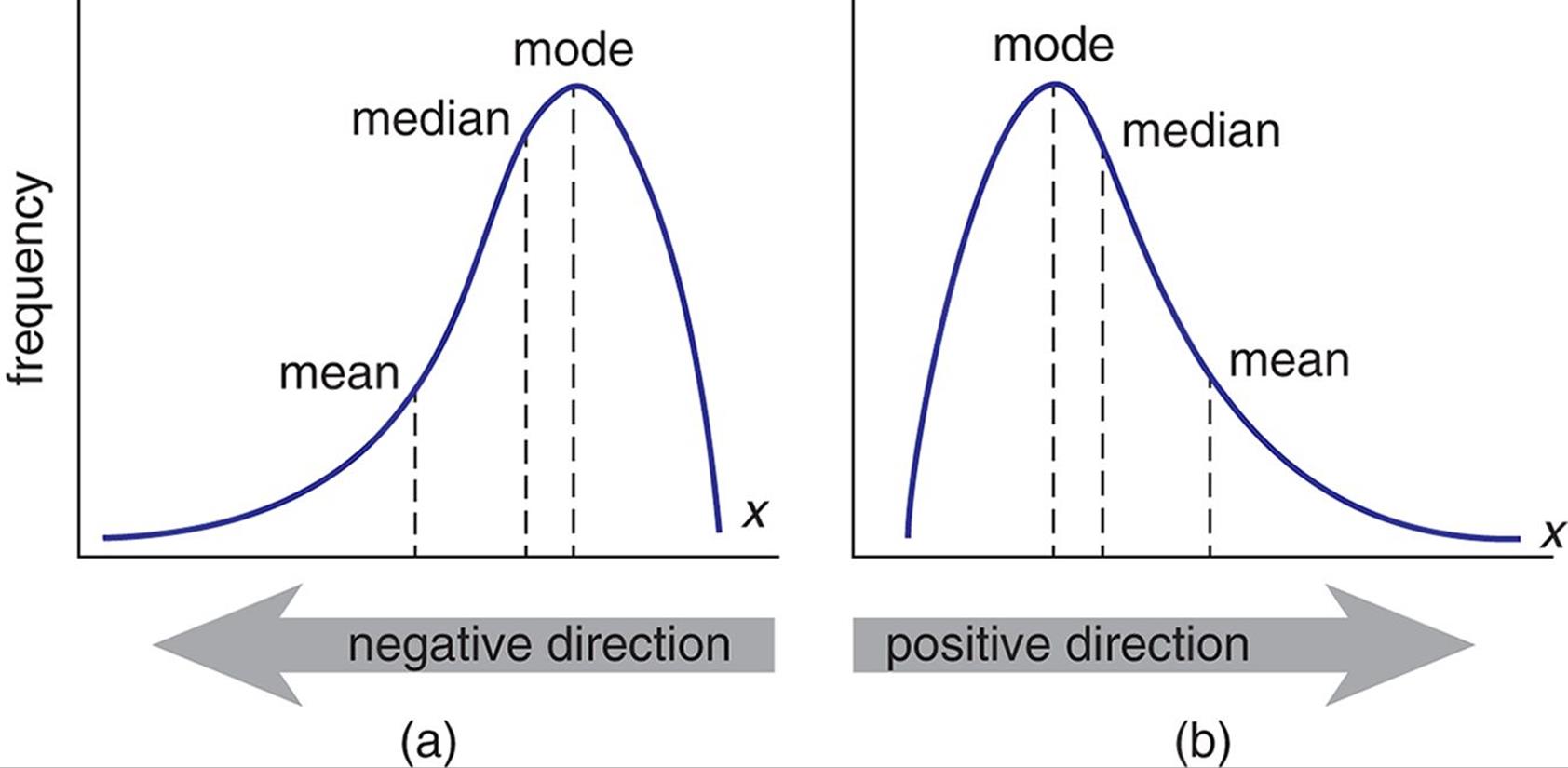
In my textbook, it states the following about skewness.

The relative position of the median to the mean of a distribution can be identified by the skewness or vice versa. If the skewness is positive, this means that it is more likely to observe a value above the mean than below the mean. Therefore, the median is more than the mean for these distributions.

Is this correct , or an error? If the median point has 50% of the probability to either side, then in a positive skew wouldn't it be more likely to observe values (of x) less than the mean? Also the median , with positive skewness, is likely to be less than the mean?

enter image description here

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ That's... wrong. The median is always in the middle, with positive skew the mean will be higher than the median, hence more values will be below the mean. $\endgroup$
    – user2974951
    Commented Sep 30, 2022 at 10:51
  • 1
    $\begingroup$ It is usually wrong, though this depends on how you define skewness. In the simplistic idea of positive skewness, the points above the median tend to be further from the median than the points below the median tend to be, so pushing up the mean. However it is possible to construct counter-examples unless you define skewness as the difference between mean and median (possibly scaled) $\endgroup$
    – Henry
    Commented Sep 30, 2022 at 12:39

0

Reset to default

Browse other questions tagged or ask your own question.