8
$\begingroup$

I am fairly new to statistics. Currently I am into (histograms) medians, arithmetic mean and all the general basics. And I came across the fact/rule that the arithmetic mean is (always) larger than the median if the distribution is skewed to the right.

Why is that?
(I would appreciate a rather simple or easily understandable answer).

Improve this question
$\endgroup$
1

3 Answers 3

Reset to default
13
$\begingroup$

A histogram represents probability by area:

Figure 1

In this figure, the white region (to the left of $x=1$) comprises half the area. The blue region comprises the other half. The boundary between them at $x=1$ is, by definition, the median: it splits the total probability exactly in half.

The areas in the next figure are shaded with varying densities of black:

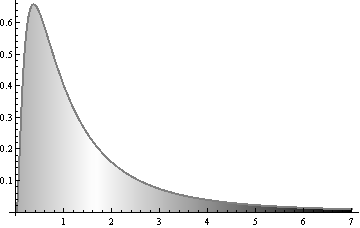
Figure 2

The density of black is directly proportional to the horizontal distance from the middle (around 1.65 here). Each point near $x=7$ is very dark. Such points contribute proportionately more to the total amount of black ink used to shade this figure. The central place (where the shading becomes white) is chosen to make total amount of black to its right equal the total amount of black to its left. This makes it equal to the mean.

We see that the distant values ($x$ larger than $3$ or so) contribute so much black that they "pull" the dividing line--the mean--towards them.

Another way to see this uses three dimensions. The mean is the point at which the two volumes (pink/yellow and blue/purple) are exactly equal:

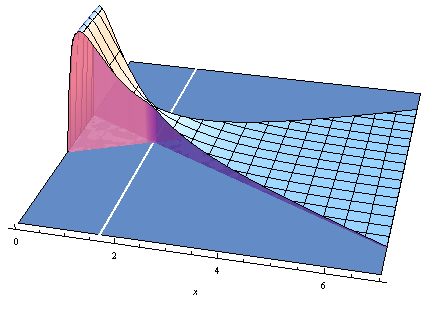
Figure 3

This figure was constructed by sweeping the original histogram (shown in the $x$ (horizontal) and $z$ (up) directions) from side to side around the mean value. This caused the long extended "tail" at the right to sweep out a larger region, because it is further from the mean than the rest of the figure. By virtue of that, it contributes more to the volume.

Were we to try the same thing by sweeping around the median (at $x=1$), we would get unequal volumes:

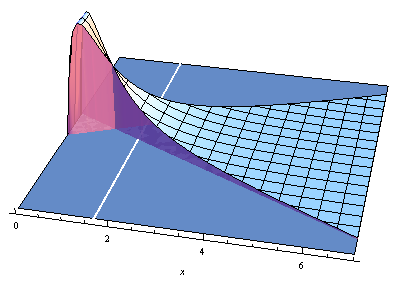
Figure 4

The white line on the ground still shows the mean, but now the axis of sweeping is around the median. Although the median correctly splits the cross-sectional area into two, it allows more volume to the right because the points to the right are "skewed" away from the median. Thus the sweeping axis has to be shifted toward larger values of $x$ to make the volumes balance.

Improve this answer
$\endgroup$
0
11
$\begingroup$

Here is a simple answer: Skew to the right means that the largest values are farther from the mean than the smallest values are (I know that isn't technically right, and not specific, but it gets the idea). If the largest values are farther from the mean they will influence the mean more than the smallest values will, thus making it larger. However, the effect on the median will be the same for the largest and smallest values.

For example, let's start with some symmetrically distributed data:

1 2 3 4 5

mean = 3, median = 3.

Now, let's skew it to the right, by making the largest values bigger (farther from the mean):

1 2 3 40 50

mean = 96/5 = 19.2 ... but median still = 3.

Improve this answer
$\endgroup$
2
  • $\begingroup$ So we can say that (on a histogram) the median describes the horizontal mean (y) and the the arithmetic mean the vertical (x)? $\endgroup$
    – daemonfire300
    Commented Mar 30, 2012 at 11:07
  • 1
    $\begingroup$ @daemonfire300 Nope. Both the median and arithmetic mean are measures of the central tendency of the distribution of the variable of interest. Usually, observed values appear on the x-axis in an histogram, with the y-axis representing frequency, counts, or density of those values grouped into bins of equal width. (In case we use unequal bin width, the area of each bin will represent the frequency, not the height of the bar.) $\endgroup$
    – chl
    Commented Mar 30, 2012 at 15:09
10
$\begingroup$

"Always" is wrong: take for example the data $\{1,1,2,2,3\}$ which has a mean of $1.8$, a median of $2$ and a positive skewness.

But more typically, positive skewness is associated with some extreme values above the median and fewer or less extreme values below the median. These will typically push up both the skewness and the mean.

Counter-examples can be constructed by having a few values far above the median on one side and more values but only moderately extreme on the other side.

Improve this answer
$\endgroup$
1
  • 4
    $\begingroup$ (+1) Excellent point. But note that "skewness" does not necessarily mean the definition in terms of the third central moment. It can be (and has been) defined in terms of the difference between the mean and median. Although that would seem to reduce this question to a tautology, it suggests that what the OP desires is an explanation for why an intuitively "skewed" distribution will tend to have its mean exceeding it median or, perhaps, why anyone would choose to call the difference between mean and median the "skewness" and attempt to relate it to visual characteristics of a histogram. $\endgroup$
    – whuber ♦
    Commented Mar 31, 2012 at 21:50

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.