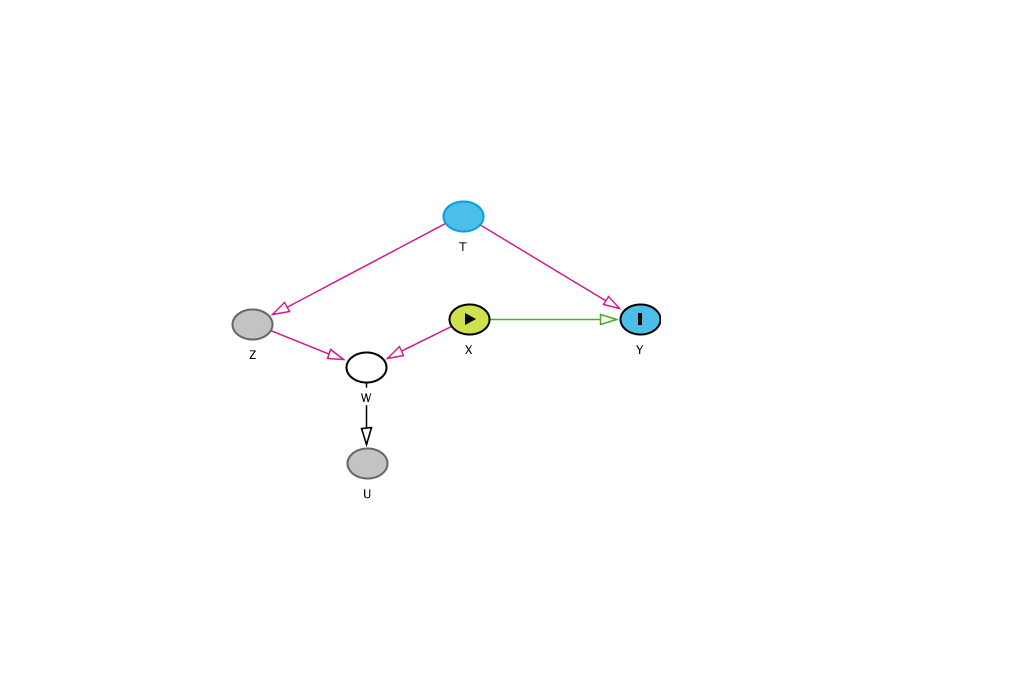
In the book Causal Inference In Statistics by Pearl, page 63, while referring to the below DAG, it says -
Thus to compute the $w$-specific causal effect, written $P(y|do(x),w)$, we adjust for $T$, and obtain
$P(Y=y|do(X=x),W=w)$ $=$ $\sum_t {P(Y=y|X=x,W=w,T=t)P(T=t|X=x,W=w)}$ (3.11)
I have the following queries -
- Why does it say - "to compute the $w$-specific causal effect, written $P(y|do(x),w)$"? Given the definition of $do(x)$ presented here, it cannot be guaranteed that $P(y|do(x),w)$ calculates the respective causal effect, when conditioning on $w$ opens up a non-causal path (highlighted in pink in the figure). Am I understanding the definition of $do(x)$ incorrectly here?
- In the equation if the summation on the right-hand side is performed,
$\sum_t {P(Y=y|X=x,W=w,T=t)P(T=t|X=x,W=w)}$
$=\sum_t {P(Y=y,T=t|X=x,W=w)}$
$=P(Y=y|X=x,W=w)$
which should not be the causal-effect as it seems to be including the association rising from the non-causal path. What am I missing here?