11
$\begingroup$

I don't know what such plots are called and thus I just gave this question a stupid title.

Let's say I have an ordered dataset as follows

4253  4262  4270  4383  4394  4476  4635  ...

Each number corresponds to the amount of postings a certain user contributed to a website. I am empirically investigating the "participation inequality" phenomenon as defined here.

In order to make it easy to grasp I would like to produce a plot which allows the reader to quickly deduce statements such as "10% of the users contribute 50% of the data". It should probably look similar to this admittedly pretty lousy paint sketch:

enter image description here

I have no clue how this is called thus I don't know where to look for. Also, if somebody had an implementation in R, that would be awesome.

Improve this question
$\endgroup$
7
  • 6
    $\begingroup$ The question is very well put (and I love the sketch). Check out ecdf in R for a start. The term is "empirical cumulative distribution function." You might also be interested in "probability plots" and "QQ plots" as well: they are versions of the ECDF showing the data on different (nonlinear) scales. $\endgroup$
    – whuber ♦
    Commented Aug 7, 2013 at 14:40
  • 7
    $\begingroup$ Lorenz curve: see en.wikipedia.org/wiki/Lorenz_curve That is easy to search for in R circles. $\endgroup$
    – Nick Cox
    Commented Aug 7, 2013 at 14:42
  • $\begingroup$ I know ecdf and have used it before, but in the "classic" way that the x-axis shows the number of postings and the y-axis their probability. I don't know how to do something like above. $\endgroup$
    – grssnbchr
    Commented Aug 7, 2013 at 14:42
  • 3
    $\begingroup$ @whuber I think "10% of the users contribute 50% of the data" is more a Lorenz curve question. A Lorenz curve is a PP plot. $\endgroup$
    – Nick Cox
    Commented Aug 7, 2013 at 14:43
  • 2
    $\begingroup$ Have a look at package ineq in R for that. $\endgroup$
    – Metrics
    Commented Aug 7, 2013 at 15:20

3 Answers 3

Reset to default
6
$\begingroup$

If you want to do it simply with the basic R commands, then following codes may help.

At first you read the data.

person<-rep(1:7)
data<-c(4253, 4262, 4270, 4383, 4394, 4476, 4635)

Then you can see the contribution of each user.

plot(person,data)
lines(person,data)

enter image description here

You can also see how much the first two, three, four, ... , seven persons contribute.

cdata<-cumsum(data)    
plot(person,cdata)
lines(person,cdata)

enter image description here

Finally you can get your desired plot (in proportions in both axes) by the following commands:

plot(person/max(person),cdata/max(cdata),xlab="Top-contributing users",ylab="Data",col="red")
lines(person/max(person),cdata/max(cdata),col="red")

enter image description here

I have labelled the axes as you wanted. It can give you a clear view about how much percentage of data are being contributed by a certain proportion of persons.

Improve this answer
$\endgroup$
0
3
$\begingroup$

I found a way to quickly visualize the Lorenz curve with ggplot2, resulting in a more aesthetic and easier-to-interpret graphic. For this latter reason, I mirrored the Lorenz curve on the diagonal line which results in a more intuitive form, if you ask me. It also contains annotation lines that should facilitate the explanation of the plot (e.g. "The 5% top contributing users make up 50% of the data"). Attention: Finding the right spot for the annotation line makes use of a quite idiotic heuristic and might not work with a smaller data set.

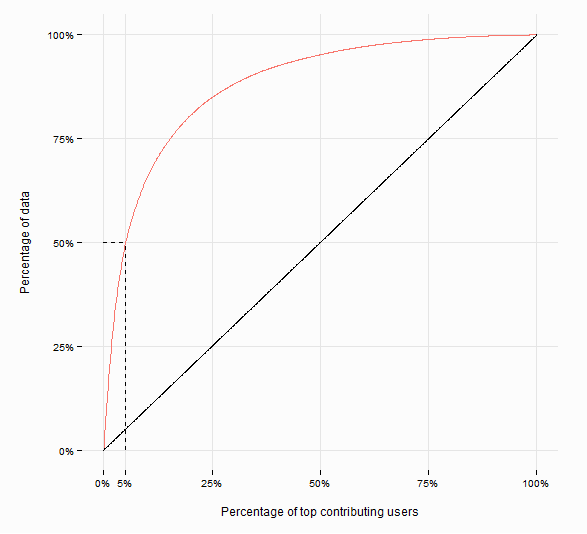
Lorenz curve (modified)

Example data:

data <- data.frame(lco = 
                     c(338L, 6317L, 79L, 36L, 3634L, 8633L, 3231L, 27L, 173L, 5934L, 
                       4476L, 1604L, 340L, 723L, 260L, 7008L, 7968L, 3854L, 4011L, 1596L, 
                       1428L, 587L, 1595L, 32L, 277L, 5201L, 133L, 407L, 676L, 1874L, 
                       1700L, 843L, 237L, 4270L, 2404L, 530L, 305L, 9344L, 720L, 1806L, 
                       35L, 790L, 1383L, 5522L, 178L, 75L, 6219L, 121L, 923L, 1123L, 
                       102L, 70L, 50L, 119L, 445L, 464L, 182L, 244L, 1358L, 7840L, 661L, 
                       70L, 132L, 634L, 4262L, 1872L, 345L, 11L, 28L, 284L, 626L, 1033L, 
                       26L, 798L, 13L, 480L, 44L, 339L, 259L, 312L, 262L, 67L, 1359L, 
                       1835L, 13L, 189L, 292L, 2152L, 215L, 39L, 1131L, 1323L, 700L, 
                       3271L, 1622L, 4669L, 125L, 281L, 277L, 232L, 1111L, 8669L, 7233L, 
                       9363L, 400L, 502L, 1425L, 904L, 2924L, 927L, 31L, 1132L, 200L, 
                       17L, 7602L, 12365L, 258L, 16L, 223L, 55L, 11L, 785L, 493L, 4L, 
                       1161L, 393L, 791L, 30L, 568L, 386L, 75L, 1882L, 674L, 29L, 4217L, 
                       332L, 103L, 332L, 30L, 168L, 277L, 176L, 49L, 19L, 76L, 144L, 
                       145L, 65L, 52L, 391L, 25L, 104L, 484L, 20L, 12L, 188L, 5677L, 
                       19L, 273L, 424L, 281L, 458L, 50L, 255L, 898L, 840L, 872L, 573L, 
                       874L, 8L, 35L, 235L, 22L, 229L, 158L, 59L, 147L, 544L, 24L, 325L, 
                       15L, 3L, 1531L, 1014L, 43L, 35L, 2176L, 934L, 253L, 69L, 784L, 
                       352L, 188L, 27L, 1516L, 322L, 1394L, 7686L, 13L, 812L, 349L, 
                       779L, 77L, 941L, 104L, 82L, 93L, 1206L, 24L, 6159L, 131L, 99L, 
                       1310L, 27L, 520L, 327L, 350L, 42L, 102L, 25L, 14L, 42L, 33L, 
                       469L, 177L, 119L, 64L, 75L, 190L, 82L, 82L, 473L, 51L, 9L, 49L, 
                       41L, 221L, 1778L, 4188L, 4L, 86L, 39L, 93L, 35L, 44L, 227L, 636L, 
                       589L, 332L, 22L, 15L, 50L, 147L, 32L, 134L, 133L, 629L, 168L, 
                       69L, 747L, 34L, 20L, 552L, 8L, 54L, 28L, 1437L, 83L, 3225L, 776L, 
                       784L, 247L, 33L, 40L, 368L, 104L, 420L, 357L, 9L, 164L, 7L, 227L, 
                       142L, 33L, 91L, 78L, 175L, 194L, 294L, 433L, 52L, 7L, 372L, 29L, 
                       220L, 371L, 375L, 233L, 12L, 35L, 795L, 35L, 43L, 50L, 57L, 32L, 
                       162L, 124L, 160L, 52L, 132L, 131L, 50L, 117L, 145L, 33L, 83L, 
                       33L, 123L, 43L, 27L, 91L, 25L, 2116L, 51L, 509L, 603L, 267L, 
                       10L, 10L, 51L, 6028L, 99L, 597L, 53L, 131L, 1084L, 1222L, 153L, 
                       70L, 746L, 437L, 82L, 299L, 1682L, 21L, 24L, 973L, 207L, 55L, 
                       116L, 47L, 48L, 149L, 100L, 690L, 129L, 80L, 1143L, 103L, 50L, 
                       242L, 708L, 316L, 83L, 61L, 15L, 203L, 435L, 474L, 47L, 718L, 
                       21L, 33L, 27L, 15L, 53L, 97L, 6L, 39L, 59L, 255L, 51L, 15L, 20L, 
                       514L, 74L, 20L, 319L, 14L, 14L, 45L, 36L, 625L, 5534L, 43L, 590L, 
                       43L, 29L, 233L, 135L, 174L, 20L, 335L, 106L, 230L, 64L, 3551L, 
                       524L, 72L, 44L, 16L, 98L, 37L, 62L, 390L, 83L, 28L, 3L, 63L, 
                       32L, 124L, 56L, 149L, 11L, 153L, 661L, 15L, 25L, 49L, 626L, 141L, 
                       38L, 23L, 123L, 530L, 47L, 6L, 18L, 222L, 391L, 71L, 75L, 234L, 
                       142L, 45L, 439L, 675L, 14L, 53L, 19L, 100L, 51L, 147L, 10L, 141L, 
                       979L, 97L, 330L, 112L, 71L, 4L, 9L, 124L, 141L, 145L, 302L, 122L, 
                       435L, 50L, 81L, 99L, 330L, 84L, 41L, 227L, 4L, 37L, 5L, 99L, 
                       210L, 7L, 183L, 67L, 98L, 157L, 96L, 150L, 22L, 288L, 391L, 188L, 
                       54L, 56L, 49L, 618L, 160L, 631L, 9L, 355L, 56L, 119L, 37L, 36L, 
                       153L, 110L, 126L, 335L, 121L, 80L, 113L, 62L, 97L, 22L, 72L, 
                       1742L, 1007L, 11L, 121L, 27L, 62L, 823L, 56L, 40L, 26L, 69L, 
                       120L, 516L, 11L, 146L, 245L, 174L, 1648L, 105L, 123L, 17L, 2565L, 
                       138L, 200L, 46L, 130L, 189L, 87L, 191L, 143L, 76L, 702L, 79L, 
                       67L, 166L, 3487L, 88L, 395L, 283L, 140L, 535L, 198L, 64L, 1033L, 
                       376L, 180L, 14L, 32L, 441L, 361L, 520L, 62L, 247L, 10L, 24L, 
                       721L, 176L, 164L, 33L, 44L, 12L, 30L, 13L, 157L, 122L, 161L, 
                       45L, 34L, 538L, 74L, 14L, 19L, 15L, 1714L, 437L, 16L, 12L, 130L, 
                       25L, 93L, 9L, 15L, 81L, 889L, 27L, 195L, 5L, 233L, 113L, 356L, 
                       51L, 146L, 6822L, 65L, 166L, 45L, 18L, 295L, 196L, 145L, 256L, 
                       14L, 8L, 89L, 32L, 20L, 239L, 68L, 63L, 21L, 102L, 158L, 1138L, 
                       48L, 113L, 144L, 83L, 93L, 3L, 1032L, 45L, 36L, 68L, 146L, 370L, 
                       25L, 10L, 290L, 858L, 19L, 17L, 64L, 42L, 38L, 711L, 144L, 58L, 
                       144L, 1736L, 188L, 38L, 58L, 91L, 255L, 58L, 307L, 4L, 9L, 60L, 
                       14L, 13L, 118L, 1549L, 108L, 483L, 34L, 1471L, 13L, 16L, 76L, 
                       163L, 147L, 75L, 520L, 4L, 59L, 73L, 32L, 24L, 656L, 16L, 2655L, 
                       38L, 20L, 1011L, 85L, 592L, 91L, 883L, 5174L, 42L, 17L, 88L, 
                       21L, 61L, 33L, 1726L, 46L, 387L, 920L, 120L, 134L, 72L, 144L, 
                       1603L, 646L, 45L, 282L, 56L, 19L, 41L, 69L, 151L, 632L, 47L, 
                       48L, 126L, 114L, 119L, 144L, 949L, 67L, 144L, 27L, 61L, 70L, 
                       287L, 64L, 323L, 27L, 149L, 1914L, 20L, 1077L, 21L, 70L, 59L, 
                       123L, 537L, 131L, 1226L, 2908L, 8L, 133L, 42L, 175L, 100L, 162L, 
                       494L, 414L, 2618L, 33L, 93L, 48L, 3676L, 553L, 705L, 58L, 268L, 
                       141L, 284L, 98L, 135L, 13L, 49L, 792L, 128L, 172L, 236L, 221L, 
                       596L, 35L, 241L, 10L, 193L, 189L, 26L, 27L, 47L, 100L, 398L, 
                       21L, 26L, 86L, 147L, 3639L, 161L, 60L, 106L, 111L, 42L, 11L, 
                       654L, 21L, 129L, 1152L, 224L, 49L, 12L, 22L, 73L, 207L, 165L, 
                       113L, 12L, 1224L, 177L, 6L, 390L, 2747L, 23L, 46L, 1166L, 805L, 
                       20L, 130L, 46L, 110L, 16L, 88L, 652L, 61L, 86L, 16L, 804L, 41L, 
                       4383L, 511L, 126L, 549L, 23L, 45L, 80L, 162L, 127L, 700L, 43L, 
                       147L, 102L, 84L, 67L, 57L, 30L, 55L, 274L, 314L, 847L, 203L, 
                       322L, 8350L, 101L, 10L, 122L, 54L, 120L, 10L, 22L, 327L, 234L, 
                       56L, 998L, 409L, 131L, 2163L, 81L, 19L, 6675L, 7L, 2182L, 1136L, 
                       71L, 15L, 286L, 133L, 132L, 37L, 144L, 28L, 392L, 870L, 312L, 
                       190L, 135L, 16L, 6L, 153L, 38L, 62L, 2710L, 36L, 61L, 37L, 88L, 
                       375L, 88L, 131L, 73L, 212L, 918L, 185L, 53L, 143L, 69L, 2231L, 
                       54L, 23L, 220L, 195L, 468L, 2009L, 364L, 54L, 277L, 1547L, 240L, 
                       1700L, 1533L, 374L, 363L, 35L, 97L, 19L, 87L, 67L, 22L, 267L, 
                       16L, 11L, 35L, 460L, 44L, 58L, 26L, 13L, 172L, 114L, 272L, 64L, 
                       254L, 49L, 440L, 329L, 48L, 93L, 10L, 70L, 17L, 120L, 5229L, 
                       118L, 133L, 43L, 2419L, 207L, 102L, 90L, 127L, 3939L, 14L, 5L, 
                       552L, 425L, 656L, 511L, 170L, 396L, 177L, 3680L, 111L, 21L, 320L, 
                       367L, 51L, 672L, 1675L, 59L, 91L, 281L, 113L, 19L, 37L, 65L, 
                       288L, 27L, 149L, 61L, 63L, 75L, 165L, 90L, 9L, 12L, 82L, 111L, 
                       157L))

Code:

# lorenz curve of user contribution
library(ineq)
library(ggplot2)
library(scales)
library(grid)
# compute lorenz curve
lcolc <- Lc(data$lco)
# bring lorenz curve in another format easily readable by ggplot2
# namely reverse the L column so that lorenz curve is mirrored on diagonal
# p stays p (the diagonal)
# Uprob contains the indices of the L's, but we need percentiles
lcdf <- data.frame(L = rev(1-lcolc$L), p = lcolc$p, Uprob = c(1:length(lcolc$L)/length(lcolc$L)))

# basic plot with the diagonal line and the L line
p <- ggplot(lcdf, aes(x = Uprob, y = L)) + geom_line(colour = hcl(h=15, l=65, c=100)) + geom_line(aes(x = p, y = p))
# compute annotation lines at 50 percent L (uses a heuristic)
index  <- which(lcdf$L >= 0.499 & lcdf$L <= 0.501)[1]

ypos <- lcdf$L[index]
yposs <- c(0,ypos)
xpos <- index/length(lcdf$L)
xposs <- c(0,xpos)
ypositions <- data.frame(x = xposs, y = c(ypos,ypos))
xpositions <- data.frame(x = c(xpos,xpos), y = yposs)
# add annotation line
p <- p + geom_line(data = ypositions, aes(x = x, y = y), 
                   linetype="dashed") + geom_line(data = xpositions, aes(x = x, y = y), 
                                                  linetype="dashed") 
# set axes and labels (namely insert custom breaks in scales)
p <- p + scale_x_continuous(breaks=c(0, xpos,0.25,0.5,0.75,1),
                            labels = percent_format()) + scale_y_continuous(
                                                                            labels = percent_format())
# add minimal theme
p <- p + theme_minimal() + xlab("Percentage of objects") + ylab("Percentage of events") 
# customize theme
p <- p + theme(plot.margin = unit(c(0.5,1,1,1), "cm"), 
               axis.title.x = element_text(vjust=-1),
               axis.title.y = element_text(angle=90, vjust=0),
               panel.grid.minor = element_blank(),
               plot.background = element_rect(fill = rgb(0.99,0.99,0.99), linetype=0)) 
# print plot
p
Improve this answer
$\endgroup$
1
  • 3
    $\begingroup$ In literature I know the majority convention by far is the reverse of that here, i.e. to exchange axes so that the curve is convex down. Inequality is a keyword here especially for finding more detailed work, especially on summarising this curve, e.g. in the study of incomes in economics. $\endgroup$
    – Nick Cox
    Commented Aug 8, 2013 at 7:22
-2
$\begingroup$

Two more ways to do this as I was recently working on this for vaccine clinical trials:

1.Use Hmisc Ecdf. This is straight forward and plots it out though bit difficult to figure out details on changing different elements of the graph.

2.Calculate cumulative distribution and then 1-cumulative is reverse cumulative. Plot the reverse using ggplot2 using geom_step if you like a step function in the graph. The function below would use ecdf from base r to give you cumulative distribution and then 1-cumulative:

     rcdf <- function (x) {
     cdf <- ecdf(x)
     y <- cdf(x)
    xrcdf <- 1-y
      }

in the above rcdf is a user-defined function defined using ecdf.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Not so. The Lorenz curve is not either the ecdf or its complement. The two axes for the Lorenz curve are both cumulative probabilities; in the case of the ecdf only one is. $\endgroup$
    – Nick Cox
    Commented Jan 14, 2016 at 19:09
  • $\begingroup$ The answer by @wnstnsmth gives a dataset and code. If you try your code on his data, you'll get quite different objects. $\endgroup$
    – Nick Cox
    Commented Jan 14, 2016 at 19:24

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.