5
$\begingroup$

I'm new here. I am planning a neuroscience experiment. I will be measuring brain signals from about twenty subjects. I will present the subjects four different kinds of stimuli. After all the data processing, I will have four power spectrums for each participant (one per stimulus type). That is, I will see the magnitude (power) of each frequency (something like this but calculated from the brain data). There is also a fifth stimulus type, which is rest (no stimulus; used as as control measure or "baseline" in neuroscience jargon).

From each of the power spectrums, I will pick two frequency bands and calculate the average power in each band. The first band is 8-12 Hz ("alpha" in neuroscience jargon) and the other 16-22 Hz ("beta" in neuroscience jargon).

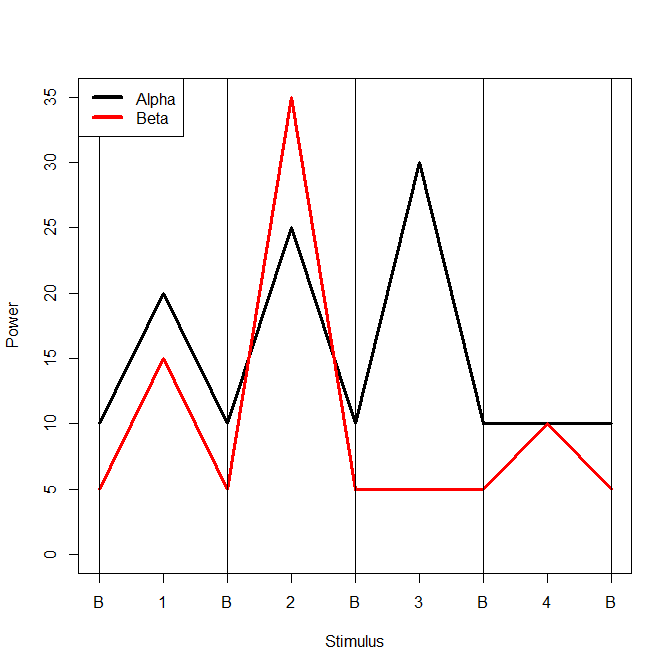
I am expecting to observe four phenomena:

  • For stimulus type 1, alpha is larger than beta. Both are larger than baseline.
  • For stimulus type 2, beta is larger than alpha. Both are larger than baseline.
  • For stimulus type 3, alpha is larger than baseline activity. Beta is equal to baseline.
  • For stimulus type 4, beta is larger than baseline activity. Alpha is equal to baseline.

How should I do my statistical tests? Is my experimental design sufficient? Note that the power of beta is always less than the power alpha in the rest state.

I am asking this because our field appears to have some problems regarding the rigour of statistical analyses (see e.g. http://www.nature.com/neuro/journal/v14/n9/full/nn.2886.html).

Improve this question
$\endgroup$
11
  • $\begingroup$ Can you make more precise predictions than "larger"? $\endgroup$
    – Livid
    Commented Apr 18, 2014 at 16:01
  • $\begingroup$ Yes. Let's say for example that the difference in power should be at least a few decibels (dB). $\endgroup$
    – neuro
    Commented Apr 18, 2014 at 16:02
  • $\begingroup$ The thing is that the patients will probably differ from session to session (unless you have data that baseline activity is very stable), so even eg Stim4 alpha==baseline alpha is not really a plausible hypothesis. You have stimulus effect + inter-individual differences + intra-individual differences + measurement error. Choosing a reasonable statistical hypothesis to test is a crucial part of the process that most people overlook. Sometimes we simply do not know enough about the system to do so, in that case exploratory analysis and parameter estimation is appropriate. $\endgroup$
    – Livid
    Commented Apr 18, 2014 at 16:17
  • $\begingroup$ Can you do all 5 stimuli in one session for each subject? Even better is to do Baseline-Stimulus1-Baseline-Stimulus2-etc. Then for each subject you should see the power spectrum change then return back to baseline. This would be very convincing even with a single subject. $\endgroup$
    – Livid
    Commented Apr 18, 2014 at 16:23
  • $\begingroup$ The power spectrums are calculated over ~10 minutes of data. The system records 1000 samples per second, so the power spectrums are calculated from 1000 * 60 * 10 = 600000 samples. (Actually, it's 1000 samples per second per channel, I average the data from 4-5 channels). There is very heavy signal processing for removing all sorts of artefacts. The intra-individual variance should be small. $\endgroup$
    – neuro
    Commented Apr 18, 2014 at 16:23

2 Answers 2

Reset to default
1
$\begingroup$

I don't have the time to write out a full length proper answer for this at the moment, so the short version of this is as follows.

An excellent book on this is "Design and Analysis of Experiments" by Montgomery, so I would strongly suggest you take a look at it for all the details. What I think you're probably looking to do is a "fractional factorial" experiment or a full factorial experiment. Your expectations will guide you in setting up the hypothesis testing.

Improve this answer
$\endgroup$
1
$\begingroup$

Am I right that your design and expected results look something like this?

enter image description here

B stands for baseline.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.