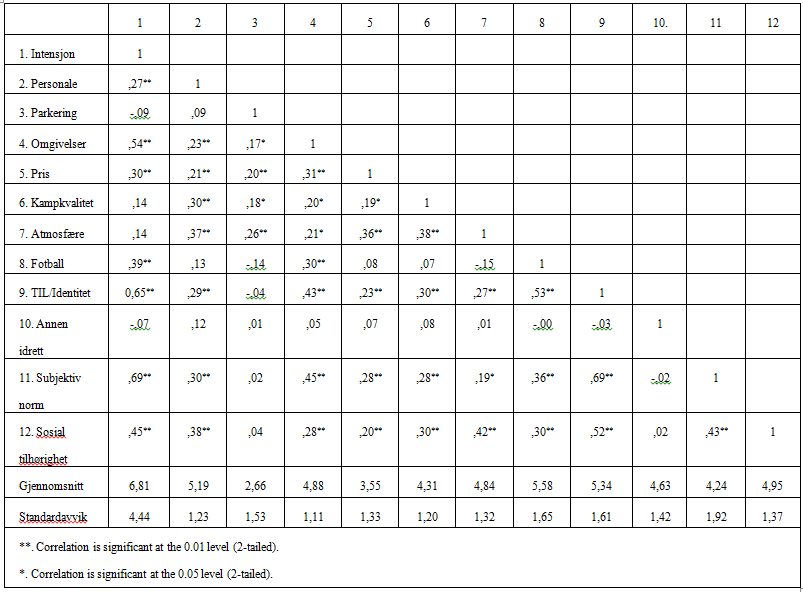
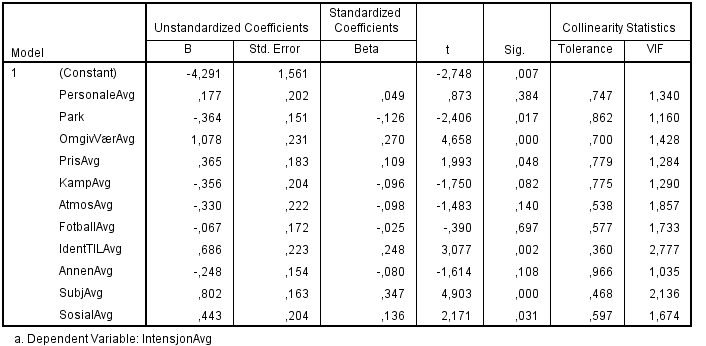
First of all, sorry for the huge pictures, but I'm in desperate need of some input and help on the results following a study. I'm trying to interpret the results from my study, but I can't quite figure out what causes some of the positive correlations to turn into negative coefficients in the regression analysis.
As you can see there are positive correlations between "atmosfære", "kampkvalitet", "fotball" and the dependent variable "intensjon". What I don't understand is how these three independent variables end up with negative coefficients in the following regression analysis. Considering VIF-values are low, which indicates low multicollinearity, I can't seem to wrap my head around this.
Some thoughts and input that might help me along the way? Much obliged!