minimum cdf given mean and variance

Question

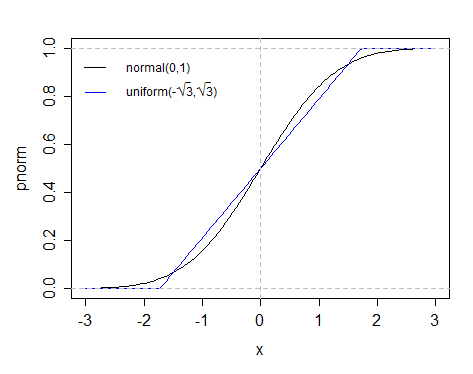
Suppose I have some distribution with mean $\mu$ and variance $\sigma^2$. How to prove that for the distribution to have min $cdf$ over all the distributions with mean $\mu$ and variance $\sigma^2$, the distribution should be normal? I want to show that the cdf for normal distribution with mean $\mu$ and variance $\sigma^2$ (i.e. $\Phi_{\mu,\sigma^2}(x)$) is lower bound for all the cdf's of distributions with mean $\mu$ and variance $\sigma^2$.

More formally, let $F(x)$ be a cdf for some distribution with mean $\mu$ and variance $\sigma^2$. I want proof that $\Phi_{\mu,\sigma^2}(x) \leq F(x) \ \forall x$

Answer 1

That this is not the case can easily be seen simply by computing the cdf for a different distribution than the normal.

So for example, consider a standard normal (i.e. let's fix $\mu$ and $\sigma$ at 0 and 1, without loss of generality$^\dagger$).

Let's try a uniform on $(-\sqrt{3},\sqrt{3})$ (which also has mean 0 and sd 1) for comparison:

We can see that it's not the case that $\Phi\leq F$ everywhere. There are places where either will exceed the other.

$\dagger$ we can do the same for the general $\mu,\sigma$ case by linearly scaling this example.

Indeed, if you're going to have the means be equal and you're going to make $F\gt\Phi$ in some region, it's going to have to be smaller somewhere else.