0
$\begingroup$

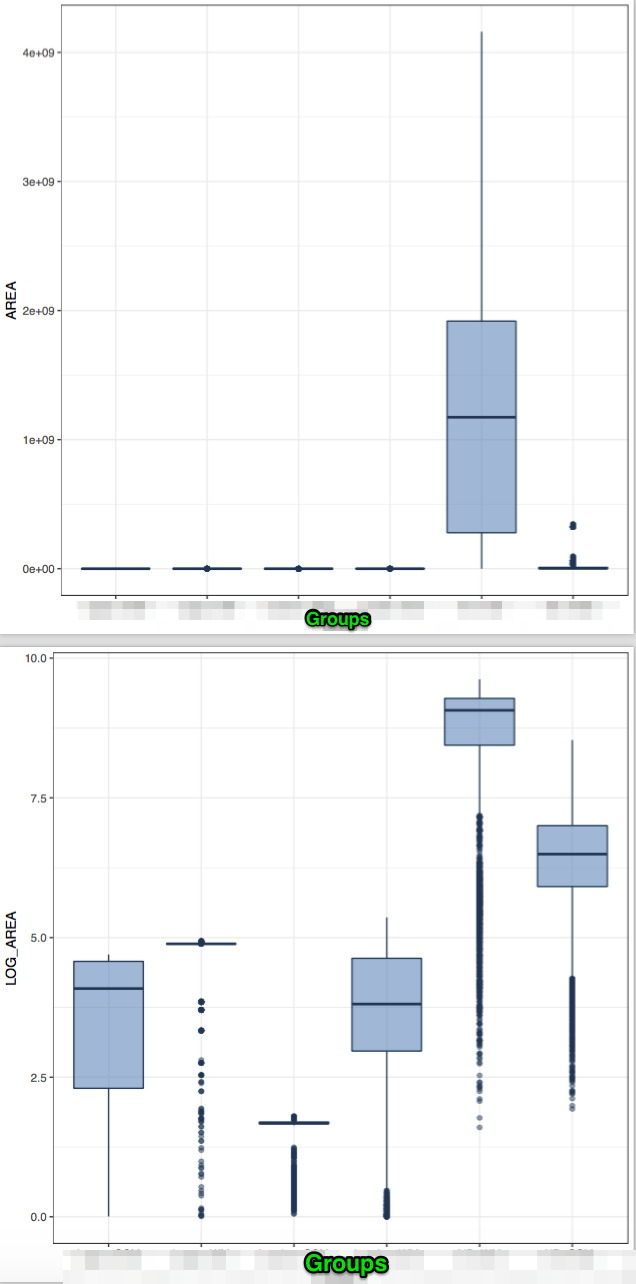
I have a set of area measurements for objects in different groups (e.g., cities), and I want to provide a visual summary of them. While Quartiles and medians are quite interpretable, I'm trying to use boxplots and I get the following plots. The first is the area measurements, and then the same values logged in base 10.

Boxplots with linear values and with logged values (log 10)

As the first plot is uninformative because of the fifth group, I decided to log the results, but I get very many low-value outliers. This is odd, as the distribution of this data is expected to be some power low, with many small values and a few large ones. I would expect outliers with high values, not with low ones.

Any suggestions on how to present this data more meaningfully?

EDIT: full dataset available here in CSV format.

Improve this question
$\endgroup$
5
  • $\begingroup$ Show the data directly for good advice. It's evidently somewhat quirky whatever you do. For example, logarithms have flipped the 5th group from right-skewed to strongly left-skewed. That's possible, but a little unusual. I note that in the simplest incarnation the log of a Pareto distribution is an exponential, and and so in that case log transformation is not expected to produce symmetric box plots. There's good reason for showing more detail when power laws are expected. $\endgroup$
    – Nick Cox
    Commented Aug 21, 2017 at 13:50
  • 2
    $\begingroup$ Since the square root of an area is a length, which could be interpretable as a proxy for city diameter, one of the first things to consider is a boxplot of the square roots of the areas. The strong negative skewness of the logs and the (possible) positive skewness of the raw data both suggest using a power somewhere between $0$ and $1$ to re-express the data. You will still see a thirtyfold range of square roots, so you will want first to examine each boxplot on its own scale before plotting them side by side. (cc @NickCox) $\endgroup$
    – whuber ♦
    Commented Aug 21, 2017 at 13:59
  • $\begingroup$ The groups don't seem similar any way when the maxima vary over several orders of magnitude. Again, we need more substantive information (than zero). Why blank out interesting detail? The data look too quirky for anyone to want to steal them. $\endgroup$
    – Nick Cox
    Commented Aug 21, 2017 at 14:05
  • 1
    $\begingroup$ The question is about a specific dataset; without access to that dataset it is hard to provide more than a few useful comments. So, voting to close as unclear (and unlikely to be helpful to any in its present form). $\endgroup$
    – Nick Cox
    Commented Aug 22, 2017 at 15:27
  • $\begingroup$ Dear all, I added a link to the dataset. $\endgroup$
    – Strabonio
    Commented Aug 23, 2017 at 16:09

1 Answer 1

Reset to default
1
$\begingroup$

Thanks for posting the data.

Following a suggestion from @whuber I focus on lengths (as likely to be better behaved).

One variable is LENGTH_M which I guess is length in metres. To avoid very large numbers on axes I divided by 1000 and call the result length in km. If that's wrong it's just a cosmetic issue for the graphs.

I then drew quantile-box plots which are quantile plots with median and quartile boxes superimposed. The results help to explain why the original box plots look rather odd but also underline how box plots can often omit illuminating detail.

I think there's a serious question about what these data mean, if anything. The gaps in distributions suggest minimally that you have a mixture of quite different entities and maximally that data quality is here compromised.

Also, from a knowledge of urban geography I know that Lagos is big, indeed very big, but that some individual area (i.e. part of the whole) is associated with a length of 689 km still seems extraordinary, regardless of how that length is defined. But this is partly a question of whether original lengths are in metres, which is where I started.

enter image description here

Improve this answer
$\endgroup$
1
  • $\begingroup$ Note: there are lots of tied values. For example, the length_m measurement 185092 occurs 403 times for Lagos_P2. You can see that on the quantile-box plots as a shelf. $\endgroup$
    – Nick Cox
    Commented Aug 23, 2017 at 18:20

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.