2
$\begingroup$

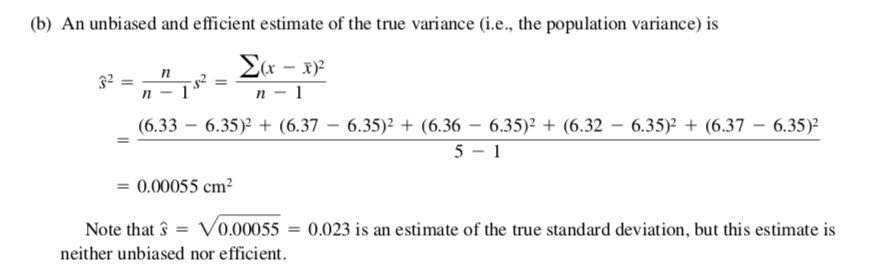
From the (excellent) Schaum's Outlines for Probability and Statistics an example from the Estimation Theory chapter shows us that the typical estimation of the variance does provide an unbiased and efficient estimation of the true variance. Yet the sqroot of that value is not so for the true standard deviation. Then .. why?

enter image description here

Improve this question
$\endgroup$
5
  • 1
    $\begingroup$ The square root is a nonlinear operation, so being unbiased doesn't carry over from the variance to the SD. $\endgroup$
    – Nick Cox
    Commented Sep 27, 2019 at 16:14
  • $\begingroup$ Thx Nick - if you have further insight and/or references pls make an answer. $\endgroup$
    – WestCoastProjects
    Commented Sep 27, 2019 at 16:15
  • $\begingroup$ Similar recent Q&A. // For normal data the bias of $S$ estimating $\sigma$ is small for moderate sample sizes. // For very small sample sizes, 'control charts' for quality mgmt often use appropriate multiples of sample range. $\endgroup$
    – BruceET
    Commented Sep 27, 2019 at 16:46
  • 1
    $\begingroup$ @BruceET Thx - upvoted that one. $\endgroup$
    – WestCoastProjects
    Commented Sep 27, 2019 at 16:50
  • $\begingroup$ The idea that unbiasedness doesn't carry over from a variance estimate to an estimate of standard deviation is correct. But I don't see how this concept applies to efficiency here. $\endgroup$
    – Michael R. Chernick
    Commented Sep 27, 2019 at 17:53

1 Answer 1

Reset to default
1
$\begingroup$

Inspired by the comment from @NickCox I found this note on Wikipedia. We have that the n-1 term does correct for the variance but the non-linear aspect of the square root allows for only partial correction on the standard deviation. There is not a general way to get an unbiased estimate of the latter. https://en.wikipedia.org/wiki/Unbiased_estimation_of_standard_deviation

enter image description here

Improve this answer
$\endgroup$

Not the answer you're looking for? Browse other questions tagged or ask your own question.