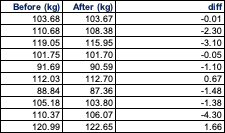
If you are looking at the weight loss (difference Before - After), then indeed the mean, standard deviation, standard error and test statistic value won't change. However, the p value - and how to interpret it depends - on what you want to demonstrate, i.e. the null / alternative hypothesis.
In your example, looking at the data it seems that we can observe weight loss in general. In order to stick to your example, we will keep the one-sample t-test approach. So, you might want to formulate your hypotheses as follows:
$H_0:$ diff = 0
$H_1:$ diff < 0
In other words, you are looking at the probability to obtain the effect observed in your sample if the null hypothesis is correct.
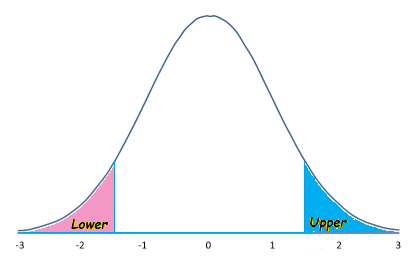
Here we are looking at a lower-tailed test, i.e. the probability that the test statistics is less than (or equal) our calculated t value (pink region in the picture below). The p-value is equal to the cumulative distribution of the test statistic (CD). In your example, p-value = 0.0379
If we set our hypotheses in the other way round:
$H_0:$ diff = 0
$H_1:$ diff > 0
then we are looking at a upper-tailed test, i.e. the probability that the test statistics is greater than (or equal) our calculated t value (blue region in the picture below). In this case, the p-value is equal to one minus the cumulative distribution of the test statistic: 1 - CD. In your example, p-value = 0.962