Comparison between regression outputs to transformed and untransformed data

Question

I want to investigate whether the values of ten dependent variables (collected in a monthly resolution) are influenced by time (in years), and, for this, I intend to perform ten linear regressions, one for each dependent variable. As not all these variables had a normal distribution, I transformed the monthly values into annual averages, which normalized the distribution of most variables, although not all. Even so, I performed the regressions with the data transformed into annual averages, and I compared the results with those of the original data regressions.

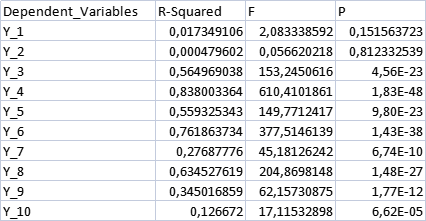
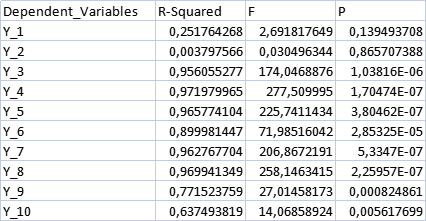
As you can see in the tables below, in both results the values of P are very similar, although the values of $R^2$ and F differ greatly in some cases. Would this difference in the values of $R^2$ and F be problematic? Would it be statistically appropriate do not transform the data into annual averages and perform regressions, even if they are not normally distributed?

• Regression output to the non-transformed data:

• Regression output to the transformed data:

Answer 1

Some regression methods, e.g. ordinary least squares, do indeed require a normal distribution. But note that it is not the data that is supposed to be normally distributed, but the errors. I.e. in the model: $$ y_i = a + b x_i + \epsilon_i $$ it is the $\epsilon_i$ that is required to be normally distributed, not the $y_i$.

Also, there are many regression models that provide for error distributions other than Gaussians. Thus, if you know that your errors are not normally distributed and those distributions cannot reasonably be approximated with Gaussians, you should check out those methods. See e.g. glm or more sophisticated R packages like brms.

In general, replacing your actual data with local averages means losing information and should be avoided.