Why do the widths of confidence & prediction intervals change across a regression line - shouldn't it be the same with i.i.d?

Question

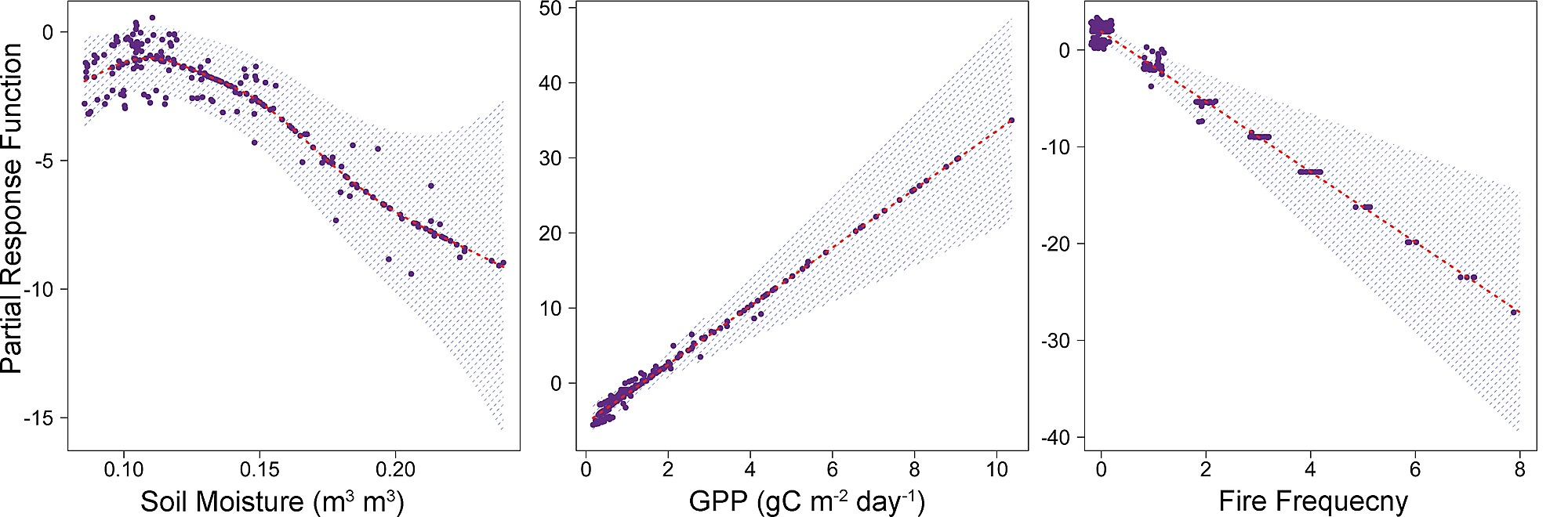
I thought that we assume i.i.d and therefore identically distributed (constant variance) over the line? But aren't the below saying that technically the distribution changes from one X value to another?

Example images of confidence intervals:

I thought that we assume i.i.d and therefore identically distributed (constant variance) over the line? But aren't the above saying that technically the distribution changes from one X value to another?

Edit

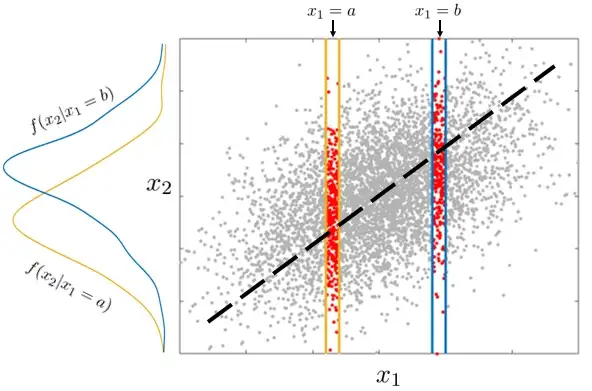
I think what I'm saying may be better described by the below image.

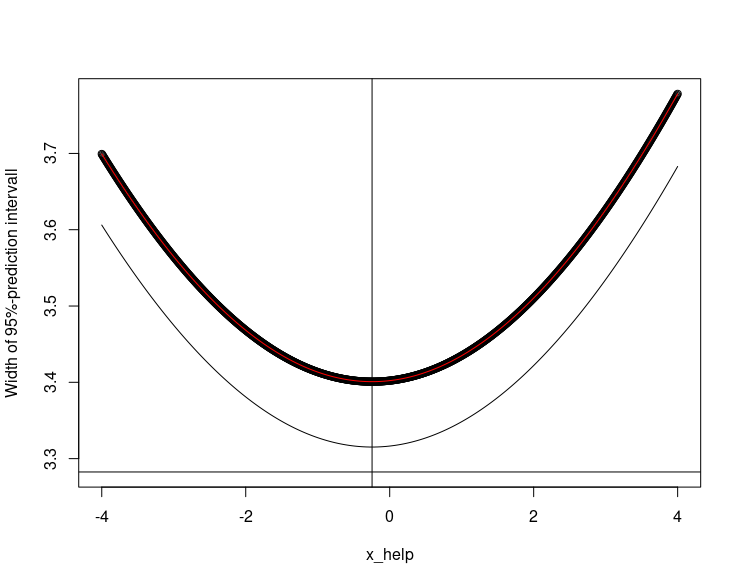
If we had enough data and could theoretically make a slice at each value of x_1, so as to obtain a theoretical conditional probability distribution of x_2 | x_1, wouldn't we expect this distribution to be the same around the best fit line for all x_1, for it to satisfy i.i.d? In the case of say normally distributed prediction intervals that widen as we go further from the centre of the data, it seems to me that we're suggesting the variance is not constant and therefore the distributions are not identical for all x.

Sorry if this makes no sense.

Answer 1

I think i can give a more mathematical answer then the two already provided.

You are fitting a linear model $Y = X\beta + \epsilon$. $X$ being a design matrix $\epsilon \sim N(0, \sigma_\epsilon)$. The estimators of your model parameters $\hat\beta$ have an estimated covariance matrix $\hat{\Sigma}_\beta$, from which the standard error of $ \hat E(Y|X=x) = \hat y(x) = x^T\hat\beta$(with $X$ now being a general stand in for the independent variables) can be calculated with $$ SE(\hat y(x)) = \sqrt{x^T\hat{\Sigma}_\beta x} $$

This will almost never be a constant, so the width of your confidence interval will vary.

Now for the predictive interval you assumed that $(Y|X=x) \sim N(\hat y(x), \hat\sigma_\epsilon)$, but you should have included the uncertainty about $\hat y(x)$, so $(Y|X=x) \sim \text{Student_t}\left(\mu = \hat y(x), \sigma =\sqrt{\hat\sigma_\epsilon^2 + SE(\hat y(x))^2}, \nu = \text{model residual df} \right)$

Here is some R code to illustrate the point:

n <- 50
x <- rnorm(n)
y <- 0.5*x + rnorm(n, sd = 1) 

m <- lm(y ~x)
x_help <- seq(-4, 4, length.out = 1000)

sum_m <- summary(m)
res.sig <-sum_m$sigma
df_conf <-  as.data.frame(predict(m, newdata = data.frame(x = x_help), interval = "conf", se.fit = T))
df_pred <- as.data.frame(predict(m, newdata = data.frame(x = x_help), interval = "predict"))
plot(x_help, df_pred$upr - df_pred$lwr, 
     ylim = c(2*qnorm(0.975)*res.sig, max( df_pred$upr - df_pred$lwr)),
     ylab = "Width of 95%-prediction intervall")
abline(v = mean(x))
# naive straight 
abline(h = 2*qnorm(0.975)*res.sig)
# adding variances, but normal distribution is wrong
lines(x_help, 2*qnorm(0.975)*sqrt(res.sig^2 + df_conf$se.fit^2))
# using t-distribution
lines(x_help, 2*qt(0.975, df = n - 2)*sqrt(res.sig^2 + df_conf$se.fit^2), col = 2) 

and here is the resulting figure:

Answer 2

Both bands are created by uncertainty in both intercept and slope.

If the intercept were a bit higher (or lower), the entire line would move up (or down).

If the slope were a bit steeper (or shallower), the line wouldn't move much in the middle of the graph, but would move a lot at the ends.

Sum those together and you get the fourth graph you included in your question. That graph shows linear regression fitting both slope and intercept. Your first graph is nonlinear regression. Your second and third are linear regression fitting only the slope but constraining the intercept to a fixed value (origin).

Answer 3

Lukas has already given a very comprehensive mathematical explanation. I just want to add a quick note about a misunderstanding in your question. The linear regression model assumes IID errors on the dependent variable $y$, for a given model $\beta$, i.e. $p(y|x,\beta)$. The prediction intervals you are looking at are most likely representative of something like the posterior predictive distribution, which provides a distribution of possible unobserved values conditional on the observed values. In other words, given some training data $(X_t,Y_t)$, the posterior predictive is the distribution of new observations, given the training data, marginalised over all possible models: $$p(y|x,X_t,Y_t) = \int_{\beta} p(y|x,\beta)\,p(\beta|X_t,Y_t)\,d\beta.$$ So, the reason the intervals are wider in some places than others is not due to the observation error (which is constant, according to the IID assumption) but rather to the larger uncertainty about the position of the regression line in those regions.

Answer 4

The intervals are informed by precision of estimates of $y|x$, so for OLS regression the estimates near the lowest and highest $x$ values are farthest away on average from all the $x$ points, and thus less precise. In the GAM case, this is somewhat ameliorated by estimation weighted towards values of $x$ local to the point being estimated, so the narrowness of the intervals follow the tightest clustering of $x$ values.