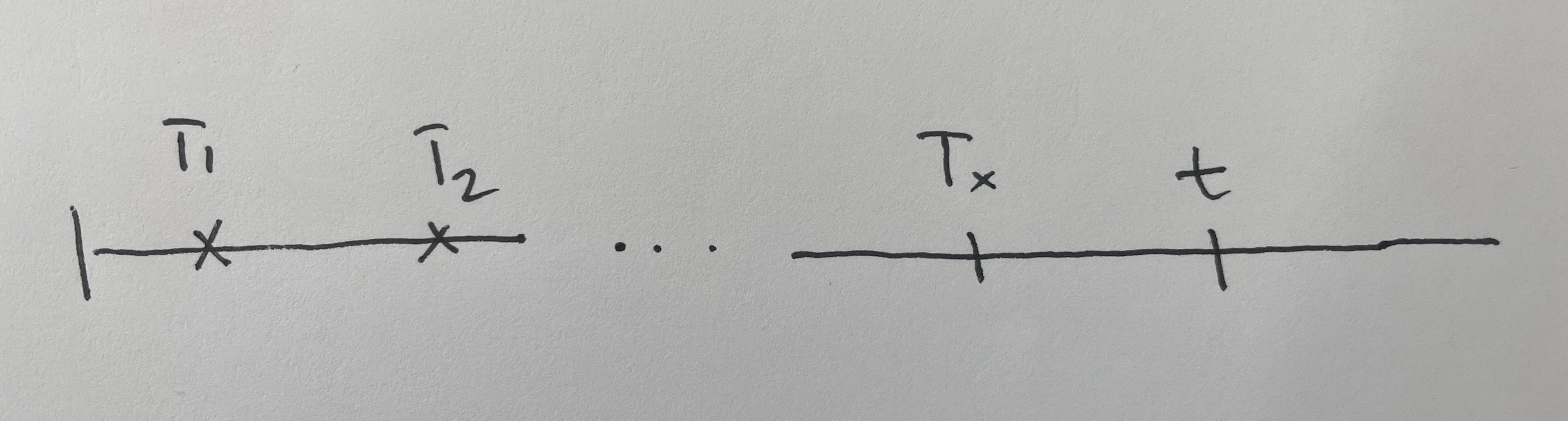0
$\begingroup$

enter image description here

This picture represents transactions. $T_1$ is the time of the first transaction, $T_2$ time of second, $T_x$ time of the $x$th transaction

$t$ is the observation period, $X(t)$ denotes the number of transactions in period $t$, $x$ is the number of transactions

Can someone help me understand the following relationship: $X(t) \geq x \Leftrightarrow T_x \leq t$

Improve this question
$\endgroup$
1

1 Answer 1

Reset to default
2
$\begingroup$

Maybe all these many $X$, $x$, $T$ and $t$'s make it look a bit confusing - consider an example: assume $t$ is time in hours, and we see the $x=5$th transaction after $t=3.2$ hours.

  • The inequality on the left hand of the relation says $X(3.2)\geq 5$, which means: at least 5 transactions have occurred within 3.2 hours.
  • The inequality on the right hand side says: $T_5 \leq 3.2$, which means that the 5th transaction occurred within 3.2 hours or earlier.

If these two sentences seem like two sides of one coin to you, that is, if they say the same: "at least 5 transactions have occurred within 3.2 hours" = " the 5th transaction occurred within 3.2 hours or earlier", then you have understood the relationship.

Improve this answer
$\endgroup$

Not the answer you're looking for? Browse other questions tagged or ask your own question.