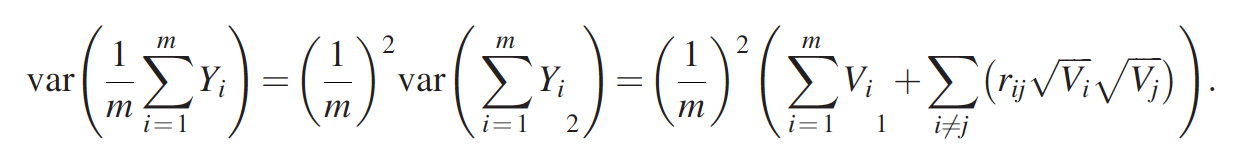1
$\begingroup$

I am confused that whether the variance used in the formula from Borenstein's method is the square of standard deviation or standard error of the mean? Could you help me? I thought it should be SD. However, at page 230 from the book (introduction to Meta-analysis) mentioned that "variance is 0.0036 and standard error is 0.06". Besides, can the formula be used to synthesize the multiple outcomes in each comparison group of the two comparison groups in each study?

enter image description here

Improve this question
$\endgroup$
6
  • $\begingroup$ Can you edit your post to show us the formula? $\endgroup$
    – mdewey
    Commented Mar 22, 2017 at 18:30
  • $\begingroup$ @Hui please indicate definitions of various terms - m, i,j and v_ i etc. $\endgroup$
    – user10619
    Commented Mar 23, 2017 at 3:50
  • $\begingroup$ indicate briefly the problem you want to solve ? $\endgroup$
    – user10619
    Commented Mar 23, 2017 at 3:53
  • $\begingroup$ In my understanding from the book, m=number of outcomes in one study, i=ith outcome, j=jth outcome, where i is not equal to j. $\endgroup$
    – Hui
    Commented Mar 23, 2017 at 4:18
  • $\begingroup$ I want to know if the study provides only mean and standard deviation, can I use the square of the SD to replace the variance term in the formula? Besides, can the formula be used for each experiment group of the two experiment groups within a study? $\endgroup$
    – Hui
    Commented Mar 23, 2017 at 4:21

1 Answer 1

Reset to default
0
$\begingroup$

Just to save this from going unanswered.

The formula gives the sampling variance of the mean which can be seen either from the formula itself (it is the variance of the sum of $m$ values of $Y$ divided by $m$) or from the fact that the value given, 0.0036, is the square of 0.06.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.