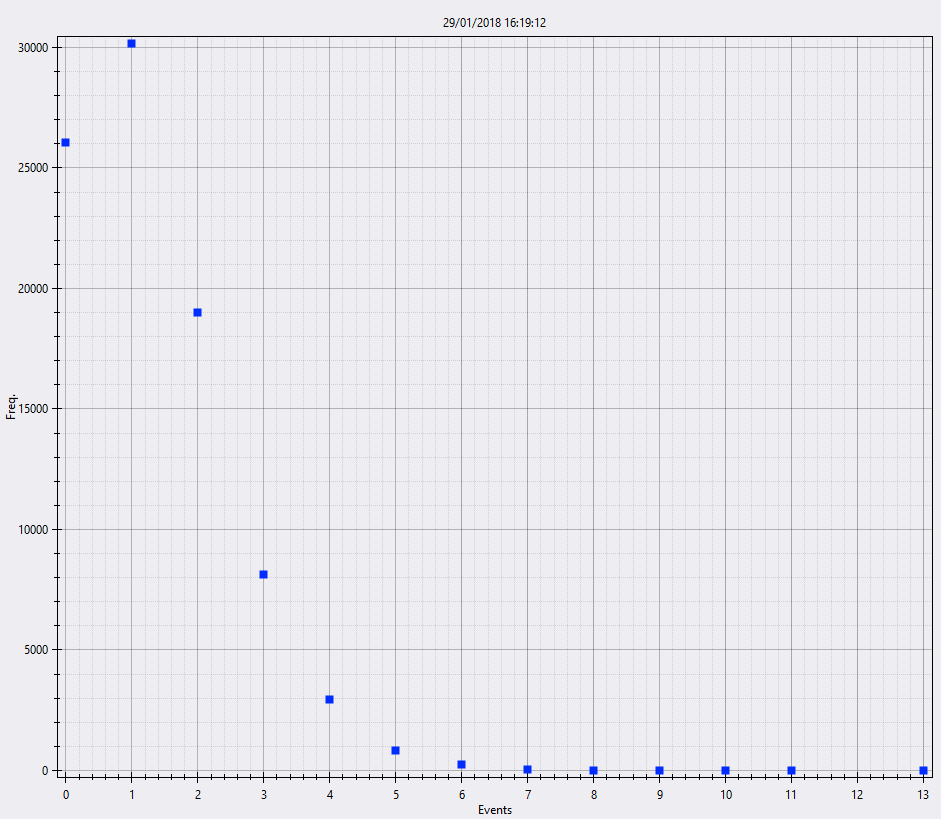
I have a experiment in which a random event that can occur $N$ times in some set period of time $T$ (where $N \in$ $\mathbb{N_0}$ but is typically less that 10 and never exceeds 100). Here I will refer to a single experiment as a "session", and for a single session we know that the probability of $n$ events occurring in some remaining time $T_{R} = T - t_{i}$ is approximately Poisson distributed, but that this distribution is not "quite right".
In order to attempt to model the exact way these events are distributed during a "session", I have run ~60,000 sessions, each one with $T = 60$ minutes. From these "sessions" I have gathered the data of how many "event" occurred in the time T. This looks like
We can model the the probability of a number of events occurring in the period $0 \leq t < T$ using a Poisson distribution (which is providing the probability of $n$ events occurring in the time-remaining $t$).
To model the events we let $t$ be the time remaining and $X_t$ be the total number of events to occur in the remaining time $t$. The model implies the estimates $P(X_t \leq c)$. So under our assumption $X_t\sim \operatorname{Poisson}(\lambda_{t})$ we have: $$ P(X_t \leq c) = e^{-\lambda}\sum_{k=0}^c\frac{\lambda_t^k}{k!}\,. $$
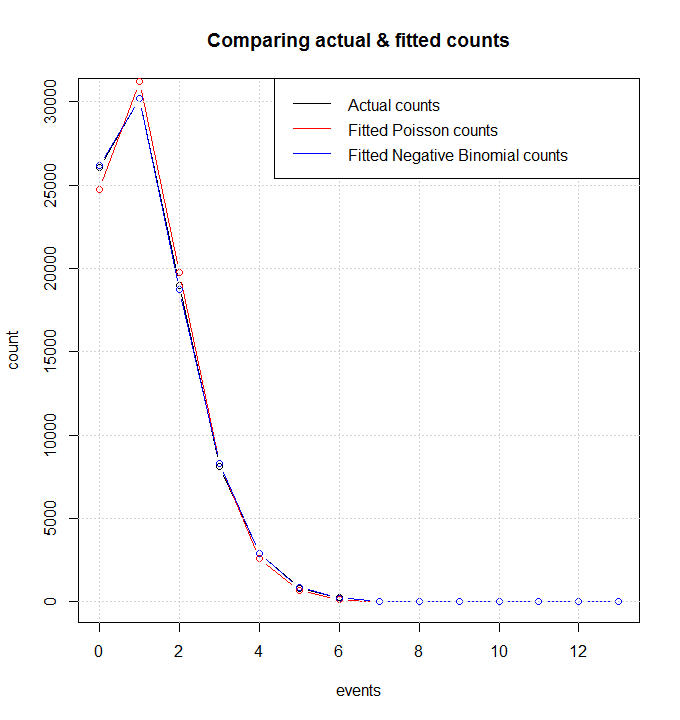
This works fairly well during simulation (where we have an implied $\lambda$ from another data source), but we have a belief that the underlying process can be more accurately modeled using a Negative Binomial distribution rather than Poisson. My problem is that I am not a statistician and I don't really know how I can go about extracting the relevant parameters for the Negative Binomial using the data above. I have access to R and am happy to write the necessary code to extract these parameters, I am just unsure about what this process/method of extraction is, can anyone outline this for me?
Better still, if we switch to use Negative Binomial distribution so that we assume now that the probability of a number of events occurring in the period $0 \leq t < T$ is $X_t\sim NB(r, p)$ and we have $$ P(X_{t} \leq c) = p^{r}\sum_{k = 0}^c (1 - p)^{k}\binom{k + r -1}{r - 1}, $$ can anyone provide an R script that can be used to extract my $r$ and $p$?
The data above is:
╔═══════════════╦══════════╗
║ Session Count ║ Events ║
╠═══════════════╬══════════╣
║ 26069 ║ 0 ║
║ 30175 ║ 1 ║
║ 18997 ║ 2 ║
║ 8136 ║ 3 ║
║ 2934 ║ 4 ║
║ 820 ║ 5 ║
║ 250 ║ 6 ║
║ 54 ║ 7 ║
║ 16 ║ 8 ║
║ 4 ║ 9 ║
║ 7 ║ 10 ║
║ 1 ║ 11 ║
║ 0 ║ 12 ║
║ 1 ║ 13 ║
╚═══════════════╩══════════╝