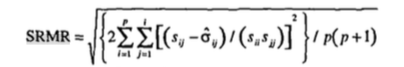2
$\begingroup$

First note, related: What is the formula for Standardized Root Mean Residual (SRMR) in the context of latent variable models (e.g., SEM, CFA)?

I was wondering what the adaption to the formula should be in case of multiple groups or longitudinal data (i.e. several time points).

enter image description here

enter image description here

As provided in the related / linked question, source: Hu, L.; Bentler, Peter (1999). "Cutoff criteria for fit indexes in covariance structure analysis: Conventional criteria versus new alternatives". Structural Equation Modeling. 6 (1): 1–55. https://dx.doi.org/10.1080%2F10705519909540118

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

For the multi group SEM, the SRMR is calculated by using a weighted average under the square root where each sample covariance matrix is compared to the model predicted covariance matrix. I did not locate a reference, but I did run a quick multi-group SEM, and then calculated the single group SEMs and confirmed this formula to be correct.

Requested Addition
Demonstration for calculating SRMR for two groups: $$SRMR = \sqrt{\frac{n_1·SRMR_1^2 + n_2·SRMR_2^2}{n_1+n_2}}$$ where $n_i$ and $SRMR_i$ are the sample size and $SRMR$ of group $i$, respectively. (Worked example in comments below.)

Improve this answer
$\endgroup$
7
  • $\begingroup$ Thanks for replying. Although I understand the idea of a 'weighted average', I am not really good at translating this into a formula. My background involves few mathematical courses. Would you mind illustrating the formula? $\endgroup$
    – Amonet
    Commented Apr 22, 2018 at 13:27
  • 1
    $\begingroup$ If you have two groups, $n_1 = 200$, $SRMR_1 = 0.04$, $n_2 = 500$, $SRMR_2 = 0.02$, then $SRMR = \sqrt{\frac{n_1SRMR_1^2 + n_2SRMR_2^2}{n_1+n_2}}=\sqrt{\frac{200·(0.04)^2 + 500·(0.02)^2}{200+400}} = \sqrt{\frac{0.52}{700}} = .0273$. $\endgroup$
    – Gregg H
    Commented Apr 22, 2018 at 14:03
  • $\begingroup$ Much appreciated, 100% clear now. Perhaps you could copy / paste this into your answer. Then I'll accept it as an answer, so other ppl will benefit from this as well :) $\endgroup$
    – Amonet
    Commented Apr 22, 2018 at 14:16
  • $\begingroup$ Happy to do so. $\endgroup$
    – Gregg H
    Commented Apr 22, 2018 at 14:18
  • $\begingroup$ Hi Gregg, I recently returned to this topic and compared the results of 4 cross-sectional CFA models (1 time point each), to a longitudinal CFA model with the 4 time points jointly. I did this in Lavaan and computed the 4 SRMRs separately and then used the formula you illustrated above (the sample sizes slightly differ due to complete case analysis where the number of missing data points differs per time point). Then I compared this number to the SRMR calculated by Lavaan based on the longitudinal model. However, the numbers are rather different: 0.06921215 vs 0.085. Any suggestions why? $\endgroup$
    – Amonet
    Commented Jun 30, 2018 at 19:44

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.