Find Probability of one event out of three when all of them can't happen together

Question

STATEMENT

Three events E, F , and G cannot occur simultaneously. Further it is known that P(E ∩ F ) = P(F ∩ G) = P(E ∩ G) = 1/3. Can you determine P(E)?

I made this diagram:

$P(E \cup F \cup G) = P(E) + P(F) + P(G) - P(E \cap F) - P(E \cap G) - P(F \cap G)$

$\implies$ $P(E) = P(E \cup F \cup G) - P(F) - P(G) + P(E \cap F) + P(E \cap G) + P(F \cap G)$

$\implies$

$P(E) = P(E \cup F \cup G) - P(F) - P(G) + \frac 13 + \frac 13 + \frac 13$

$\implies$

$P(E) = P(E \cup F \cup G) - P(F) - P(G) + 1$

Now what to do next?

Looks like this diagram matches better with the problem description:

Answer 1

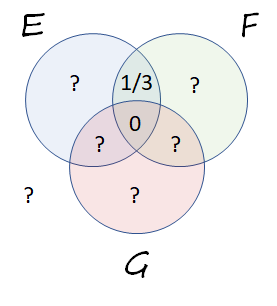
This Venn diagram displays a situation where the chance of mutual intersection is zero:

From $\Pr(E\cap F) = 1/3$ we deduce all this probability lies in the overlap of the $E$ and $F$ disks, but not in the mutual overlap of all three disks. That permits us to update the diagram:

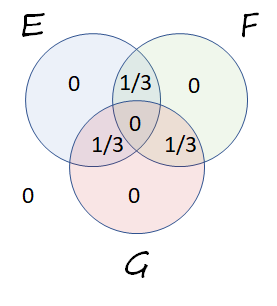
Applying the same reasoning to $\Pr(F\cap G) = \Pr(E\cap G) = 1/3,$ we obtain a Venn diagram displaying all the information in the question:

The Axiom of Total Probability asserts the sum of all the probabilities (including the probability for the complement of $E\cup F\cup G,$ shown at the bottom left) is $1.$

An even more basic probability axiom asserts all probabilities must be non-negative. But since $1/3+1/3+1/3+0=1,$ all the possible probability already appears. The remaining probabilities must be zero, meaning the picture can be completed only like this:

Finally, a third axiom (the same one used in the second step of filling in the Venn diagram) asserts the probability of $E$ equals the sum of the probabilities of its four parts, because they are disjoint. Thus, beginning with the central probability and moving counterclockwise around the disk that portrays $E,$

$$\Pr(E) = 0 + 1/3 + 0 + 1/3 = 2/3.$$

---

One moral worth remembering:

Draw Venn diagrams in full generality so they show all possible intersections of the sets, even when you know some of the probabilities are zero.

This helps you keep track of all the information systematically. (It's also conceptually more accurate, because sets of probability zero do not have to be nonempty!)

Answer 2

If you try to fill in the Venn diagram, you can't put non-zero entries inside regions other than represented by pairwise intersections. They'll form up the sample space by themselves, which means $$\mathbb P(E)=\mathbb P(E\cap F)+\mathbb P(E\cap G)=2/3$$

Answer 3

The answer to the question "Can you determine $P(E)$?" is Yes.

Given events $E, F, G$ defined on a sample space $\Omega$, we know that \begin{align} &E\cap F\cap G\\ &E\cap F\cap G^c\\ &E\cap F^c\cap G\\ &E\cap F^c\cap G^c\\ &E^c\cap F\cap G\\ &E^c\cap F\cap G^c\\ &E^c\cap F^c\cap G\\ &E^c\cap F^c\cap G^c\\ \end{align} are $8$ mutually exclusive events whose union is $\Omega$. Thus, the sum of the probabilities of these $8$ events is $1$. Now, we are told that $E, F, G$ cannot occur simultaneously, that is, $E\cap F\cap G = \emptyset$ and so $P(E\cap F\cap G) = 0$. We are also told that \begin{align} P(E\cap F) &= P(E\cap F\cap G) + P(E\cap F\cap G^c) = \frac 13\\ P(E\cap G) &= P(E\cap F\cap G) + P(E\cap F^c \cap G) = \frac 13\\ P(F\cap G) &= P(E\cap F\cap G) + P(E^c\cap F \cap G) = \frac 13 \end{align} where we can feel comfortable about the sum in the middle in each equation by musing on the fact that the probability of the union of two mutually exclusive events is the sum of the probabilities of the two events. Since $P(E\cap F\cap G)=0$, we conclude that \begin{align}P(E\cap F) &= P(E\cap F\cap G^c) = \frac 13\\ P(E\cap G) &= P(E\cap F^c \cap G) = \frac 13\\ P(F\cap G) &= P(E^c\cap F \cap G) = \frac 13 \end{align}

But, of the $8$ mutually exclusive events listed above whose union is $\Omega$, we have identified three events whose probabilities add up to $1$ and so the other $5$ events (one of which is $E\cap F\cap G$) must have probability $0$. Consequently, \begin{align} P(E) &= P(E\cap F\cap G) + P(E\cap F\cap G^c) + P(E\cap F^c\cap G) + P(E\cap F^c\cap G^c)\\ &= 0 + \frac 13 + \frac 13 + 0\\ &= \frac 23 \end{align} By symmetry (or by a brute force repetition of the above arguments mutatis mutandis), we can conclude that $E, F, G$ all have probabiity $\frac 23$.

Answer 4

Can we think of it that way?

P(E ∩ F ) = P(F ∩ G) = P(E ∩ G) = 1/3

P(E ∩ F ) + P(F ∩ G) + P(E ∩ G) = 1

Meaning that The probability of event E happening by itself is zero, which means it can only happen with either F or G and it can't happen with both.

P(E) = P(E ∩ F ) + P(E ∩ G) = 1/3 + 1/3 = 2/3

Answer 5

Since the events $(E,F)$, $(E,G)$ $(F,G)$ are mutually exclusive and sum to one we can use the law of total prob: $$ P(E) = P(E, F) + P(E, G) = \tfrac{2}{3} $$ Since $P(E \mid E,F)P(E, F) = P(E, F)$, ditto for $E,G$ and $P(E \mid F, G) = 0$.