When sampling K numbers out of 1,...,N with replacement, and calculating the histogram of the sampled set - what's the distribution? Or if that's to hard, whats the expectation of each bin in the histogram?
Ex: Sampling K=5 out of N=3, we could get the set 2,2,2,3,3 in which case the histogram would be 0,3,2. The histogram has N elements, and I'm asking how each of them distributes.
Let $N = 5.$ Then you are simulating the discrete uniform distribution on the integers 1 through $5,$ each with probability $1/5.$
With $K = 1000$ values sampled from among $1$ through $5,$ you ought to get an idea that the simulation process produces values that match this discrete uniform distribution. [I'm using R, following @Fiodor1234's Comment to use a larger $K.]$
set.seed(413) # for reproducibility of results
x = sample(1:5, 1000, rep=T) # sampling 1000 values at random
summary(x); length(x); sd(x)
Min. 1st Qu. Median Mean 3rd Qu. Max.
1.000 2.000 3.000 3.017 4.000 5.000
[1] 1000 # sample size
[1] 1.3837 $ sample standard deviation (aprx 1.414).
table(x)
x
1 2 3 4 5
180 219 194 218 189
Distribution:
j = 1:5; pdf = rep(1/5, 5) $ PDF of discrete unif dist'n
$$P(X=1) = P(X=2) = P(X=3) = P(X=4) = P(X=5) = 1/5.$$ Thus $$E(X) = \frac{1}{5}\cdot 1 = \frac{1}{5}\cdot 2 + \cdots + \frac{1}{5}\cdot 5 = \frac{15}{5} = 3,$$ $$Var(X) = E[(X-3)^2] = \frac{1}{5}\left[(-2)^2 + (-1)^2 = 0^2 + 1^2 + 2^2\right] = \frac{10}{5} = 2,$$ and $SD(X) =\sqrt{2} = 1.414.$
sum((1/5)*j)
[1] 3 # E(X)
sum((1/5)*(j-3)^2)
[1] 2 # Var(X)
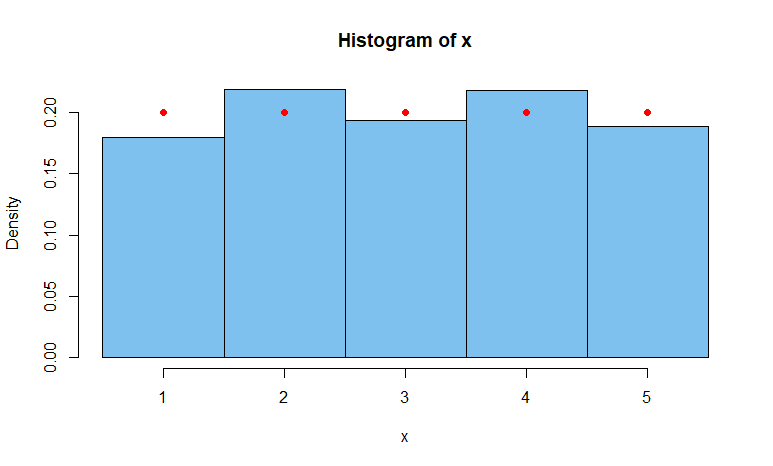
Make a histogram of this sample of 1000 values of $X$ and overlay red dots to show the exact distribution of $X.$
cutp = 0:5 + .5 # boundaries of histogram bins
hist(x, prob=T, br=cutp. col="skyblue2")
points(j, pdf, pch=19, col="red")
