26
$\begingroup$

I ran across this density the other day. Has someone given this a name?

$f(x) = \log(1 + x^{-2}) / 2\pi$

The density is infinite at the origin and it also has fat tails. I saw it used as a prior distribution in a context where many observations were expected to be small, though large values were expected as well.

Improve this question
$\endgroup$
5
  • $\begingroup$ out of curiousity, have you got a citation for the source where you saw this originally? $\endgroup$
    – JMS
    Commented Feb 10, 2011 at 20:49
  • 2
    $\begingroup$ JMS: "The horseshoe estimator for sparse signals" by Carvalho, Polson, and Scott. I saw it as a preprint, but it may have been published in Biometrika by now. They don't exactly use this prior, but the density above is an approximation to a special case of their prior. $\endgroup$
    – John D. Cook
    Commented Feb 10, 2011 at 21:11
  • 2
    $\begingroup$ It's been published: dx.doi.org/10.1093/biomet/asq017. $\endgroup$
    – fabians
    Commented Feb 15, 2011 at 15:39
  • $\begingroup$ Which special case are you approximating? I've read it, but can't really relate your expression to the expressions given in the paper...? $\endgroup$
    – fabians
    Commented Feb 15, 2011 at 15:44
  • 1
    $\begingroup$ @fabians: The case I had in mind was sigma^2 = tau^2 = 1 in Theorem 1. It says the horseshoe density is bounded above and below by multiples of log(1 + c/x^2). So maybe the distribution I mentioned above is more of a simplification of the horseshoe density than an approximation. $\endgroup$
    – John D. Cook
    Commented Feb 16, 2011 at 13:54

2 Answers 2

Reset to default
16
$\begingroup$

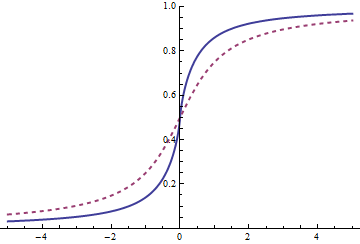
Indeed, even the first moment does not exist. The CDF of this distribution is given by

$$F(x) = 1/2 + \left(\arctan(x) - x \log(\sin(\arctan(x)))\right)/\pi$$

for $x \ge 0$ and, by symmetry, $F(x) = 1 - F(|x|)$ for $x \lt 0$. Neither this nor any of the obvious transforms look familiar to me. (The fact that we can obtain a closed form for the CDF in terms of elementary functions already severely limits the possibilities, but the somewhat obscure and complicated nature of this closed form quickly rules out standard distributions or power/log/exponential/trig transformations of them. The arctangent is, of course, the CDF of a Cauchy (Student $t_1$) distribution, exhibiting this CDF as a (substantially) perturbed version of the Cauchy distribution, shown as red dashes.)

enter image description here

Improve this answer
$\endgroup$
9
  • 5
    $\begingroup$ @whuber, note that $-2\log(\sin(\mathrm{arctan}(x))) = \log(1+x^{-2})$, which relates the form of the cdf closer to that of the pdf. It's also interesting to note that this pdf is asymptotic to one-half the pdf of a standard Cauchy. So, the main reason for its use would seem to have to be for its behavior around 0. $\endgroup$
    – cardinal
    Commented Feb 10, 2011 at 3:41
  • 1
    $\begingroup$ @whuber, though I think I see where you are coming from with regard to your statement about cdfs having closed forms (hint: Louiville), I would urge caution with that remark. The Cauchy distribution itself is a "counterexample" in that respect. $\endgroup$
    – cardinal
    Commented Feb 10, 2011 at 3:54
  • 1
    $\begingroup$ @whuber, well perhaps I would have been better off asking for clarification rather than assuming. What was your point regarding your comment that a closed form cdf severely limits the possibilities? $\endgroup$
    – cardinal
    Commented Feb 10, 2011 at 12:27
  • 1
    $\begingroup$ @cardinal I am performing a wide search in the sense of finding a named (or heretofore studied) distribution $G$ and a relatively simple re-expression $y$ (such as a power or logarithm etc.) such that $y(X)$ has cdf $G$ iff $X$ has pdf $f$. If a distribution has been studied before, then it's highly likely its CDF has been obtained and, if it can be written in closed form, that form has also been published. Therefore we need only look for functional forms $G$ that look like $u - \tan(u)\log(\sin(u))$ with $u = u(x)$. Know of any? $\endgroup$
    – whuber ♦
    Commented Feb 10, 2011 at 13:54
  • 1
    $\begingroup$ @whuber, sorry, yes. My attempt to clarify something I was saying mucked things up. My apologies. And, yes, I noticed the fact that it couldn't be a mixture right before your most recent comment. But, you beat me to it! $\endgroup$
    – cardinal
    Commented Feb 10, 2011 at 14:56
6
$\begingroup$

Perhaps not.

I could not find it in this fairly extensive list of distributions:

Leemis and McQuestion 2008 Univariate Distribution Relationships. American Statistician 62(1) 45:53

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.