1
$\begingroup$

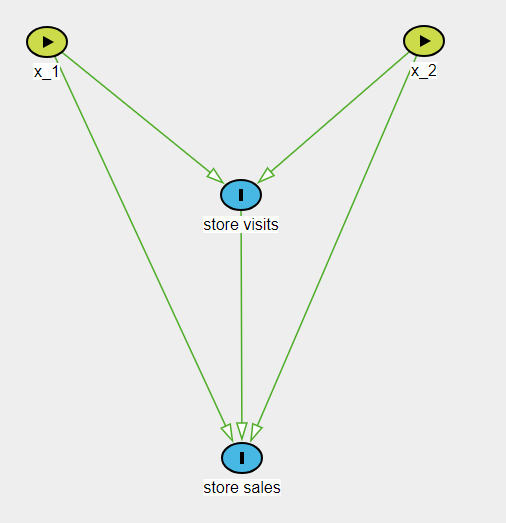
I would like to understand some intuitions behind the following causal graph/SCM.

enter image description here

Where as $X_1, X_2$ are expenditure on promotional activities. My main interest lies in understanding the fact that even though people HAVE to visit the store to buy something(generate a store sales) $X_1, X_2$ can also have an effect directly on $store \ sales$ beyond their effect on $store \ visits$.

E.g $X_1$ may generate a large amount of store visits but the customers arriving to the store are not eager to buy anything, on the other hand $X_2$ might bring few customers to the store but the ones that arrive buy a lot of items.

Thus i am trying to justify intuitively/examplify why we need the direct effect from $X_1$ to $store sales$ when we keep the direct effect $X_1$ to $store visits$. Bare in mind that customers have to visit the store in order to purchase.

I.e my intuition is that they may affect store visits differently from how they affect store sales, would this be a valid interpretation?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

Let's forget $X_2$ for a second. The structural causal model for $\text{sales}$ is $\text{sales} = f(X_1, \text{visits})$. That comes from reading the graph and is all you can say about the causal relationships from the graph. Your detailed explanation about $X_1$ modifying the effect of $\text{visits}$ on $\text{sales}$ does not come from the graph; that is a specific model $f(.)$ that is left unspecified by the graph and is separate from the general nonparametric structural causal model.

It sounds like you think there is a specific relationship that you are interested in observed or testing, which is that when $\text{visits} = 0$, $\text{sales} = 0$, but when $\text{visits} \ne 0$, $\text{sales}$ is a complex function of both $\text{visits}$ and $X_1$, where the relationship between $\text{visits}$ and $\text{sales}$ depends on the value of $X_1$. Such a model might look like this: $$ \text{sales} := I(\text{visits} > 0) \times g(\text{visits}, X_1) $$ where $g(.,.)$ is some function that describes the complex relationships and $I(.)$ is an indicator function that is 1 when its argument is true and 0 otherwise. An example of $g(.,.)$ could be something as simple as a linear model with an interaction: $$ \text{sales} := I(\text{visits} > 0) * (\beta_0 + \beta_1 \text{visits} + \beta_2 X_1 + \beta_3 \text{visits}\times X_1) $$ Again, that is a specific model that cannot be read from this or any DAG (because DAGs are inherently nonparametric and general).

Note that the relationship between $X_1$ and $\text{visits}$ is irrelevant here; your question seems to be about defining causal relationships when under some circumstances that relationship is absent but other times is present and interactive.

Improve this answer
$\endgroup$
5
  • $\begingroup$ thanks for answering, it is obvious that my intention with the post was not clear, sorry. I am just interested in the intuition/examples of why we would construct such a causal graph where we have some sort of driver, $x_1$ lets say that affects people going to the store(direct effect) but also affect store sales(direct effect) but we also have the indirect affect(x_1 -> store visit -> store sales) and the intuition behind such an representation. I.e even though people have to visit the store, we still need the direct effect x_1 -> store sales to account for what? $\endgroup$
    – jack
    Commented Sep 19, 2023 at 18:55
  • $\begingroup$ Thus i am trying to justify intuitively/examplify why we need the direct effect from $X_1$ to $store sales$ when we keep the direct effect $X_1$ to $store visits$. Bare in mind that customers have to visit the store in order to purchase. i'll accept and upvote after some small examples/affirming my intuition behind why such a formulation would make sence. I'll try to edit the post to make it more clear aswell. $\endgroup$
    – jack
    Commented Sep 19, 2023 at 18:56
  • 1
    $\begingroup$ Well, I think I kind of answered it. If $X_1$ changes the way $\text{visits}$ is related to $\text{sales}$, then $X_1$ is part of the causal model for $\text{sales}$ and the first strucutral model I proposed stands. If you think that only way $X_1$ is related to $\text{sales}$ is through $\text{visits}$, and $X_1$ doesn't change the relationship between $\text{visits}$ and $\text{sales}$ (i.e., it just changes the value of $\text{visits}$), then the correct causal model for $\text{sales}$ would be $\text{sales} := f(\text{visits})$. $\endgroup$
    – Noah
    Commented Sep 19, 2023 at 19:25
  • $\begingroup$ i think i am getting stuck in the thoughtprocess that just because customers have to visit the store in order to generate sales it doesnt mean that some variation in $store \ sales$ cannot solely be explained by $store \ visits$, this is why i tried to exemplify this by "E.g X1 may generate a large amount of store visits but the customers arriving to the store are not eager to buy anything, on the other hand X2 might bring few customers to the store but the ones that arrive buy a lot of items." which is the reason behind direct edges from $x_1, x_2$ to store sales $\endgroup$
    – jack
    Commented Sep 20, 2023 at 11:50
  • $\begingroup$ Yes, what you are describing is exactly effect modification/moderation combined with mediation, which is represented by direct arrows from $X_1$ to $\text{sales}$ and to $\text{visits}$. My answer here gets at that a bit, too. The DAG is consistent with your substantive theory, but the DAG is far less specific than your theory, which proposes specific mechanisms defined by functions. In my answer I tried to give examples of the specific functions, all of which are compatible with the DAG. $\endgroup$
    – Noah
    Commented Sep 20, 2023 at 15:53

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.