5
$\begingroup$

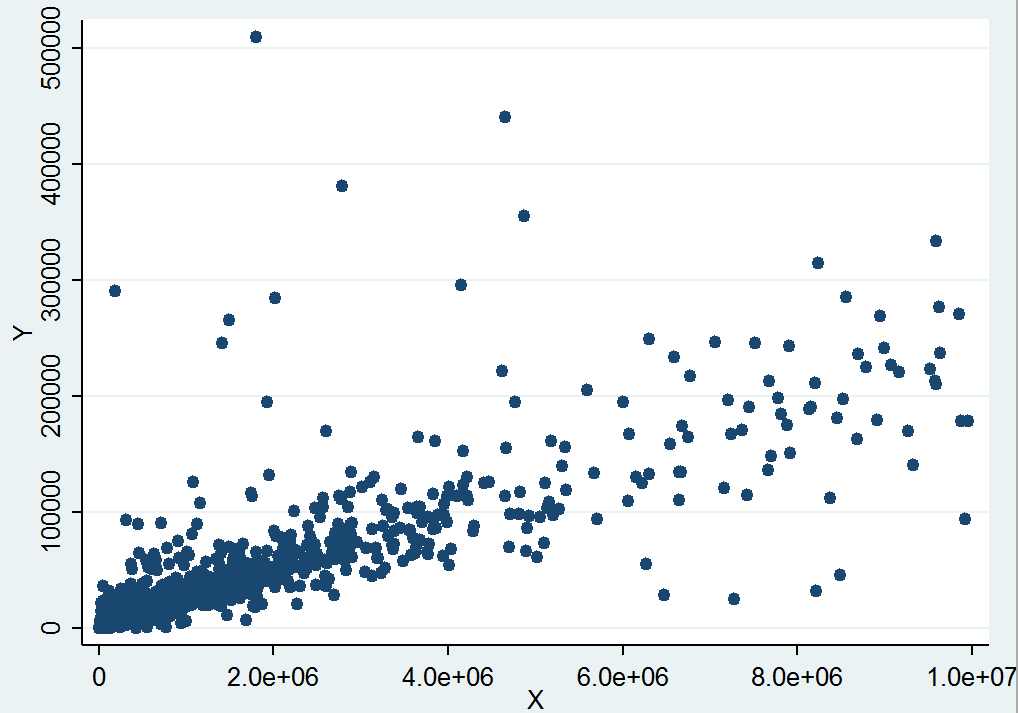
I have a simple linear regression model and am trying to locate structural breaks in the relationship between the two variables. The data are cross-sectional, not time-series.

I've been using Wald tests on pairs of dummies (1 if X is above some threshold, zero otherwise) & interaction terms (of the dummy and X) and found quite a few "candidate" points. But after reading up on the topic, it seems this method is frowned upon. That is, testing multiple points in hopes of finding something rather than testing a "known" point.

The estat sbsingle command in Stata shows some promise, but requires a gapless time series. Is it advisable/reasonable/defensible to assign ranks to the observations based on each's X value, and then treat the rank as a time-series variable in order to meet the estat command's requirements?

Here is the scatter plot of my data:

scatterplot

Improve this question
$\endgroup$
5
  • $\begingroup$ Can you post an example scatterplot of your data? $\endgroup$
    – GeoMatt22
    Commented Aug 31, 2016 at 0:29
  • 1
    $\begingroup$ You might find this post relevant. $\endgroup$
    – dimitriy
    Commented Aug 31, 2016 at 0:47
  • $\begingroup$ Does a Chow test not work here? $\endgroup$
    – VCG
    Commented Aug 31, 2016 at 13:29
  • $\begingroup$ My understanding is that a Chow test, which is essentially what I was doing with the shotgun-approach Wald tests, is best used when there is a "known" break to be tested as opposed to when one is looking somewhat blindly for which points may qualify as breaks. $\endgroup$
    – Andy_3000
    Commented Aug 31, 2016 at 13:50
  • $\begingroup$ Try a Log-Log transform, the correlation will improve. $\endgroup$
    – Carl
    Commented Apr 16, 2017 at 10:44

2 Answers 2

Reset to default
1
$\begingroup$

I think a discontinuity would meet your definition of a structural break in an X-Y relationship. Check out 'regression discontinuity' and see what you think.

Improve this answer
$\endgroup$
0
$\begingroup$

yes, it must be time series. Once I raised a question in a workshop. If we have IRS, CRS and DRS between labor any output , then we can detect structural break in this cross sectional data. An econometrician there said, it is time series not cross sectional as both labor and output are time-series.

Improve this answer
$\endgroup$
1
  • 4
    $\begingroup$ Given that "time series" and "structural break" have a variety of definitions and interpretations, to avoid misconceptions and error it is important to state what you understand these terms to mean, because this answer does not appear to be correct for many reasonable interpretations. $\endgroup$
    – whuber ♦
    Commented Jan 16, 2019 at 14:03

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.