I think I'm overthinking it, but this p-value for the exp.c:anx.c interaction (3.84e-09) is significant, right? In other words, it's much smaller than 0.001? Irrational numbers are confusing for me, and I also have a negative t-value that is throwing me off.
I'm also confused at the bottom where it shows: p-value: < 2.2e-16, what does this mean? Overall for the model?
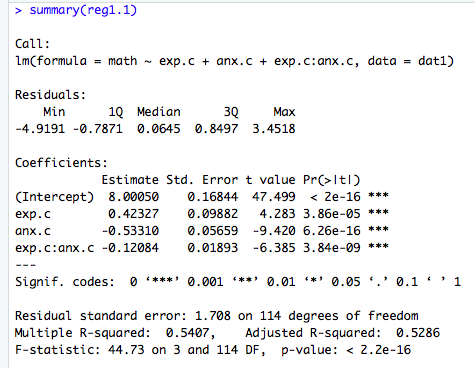
p-value for the exp.c:anx.c interaction (3.84e-09) is significant, right?
Yes, at the typical $\alpha=0.05$ level (i.e. $p=3.8 \times 10^{-9} \ll 0.05$) ($\ll$ means "much less than")
In other words, it's much smaller than 0.001?
Not sure what you mean here. Do you mean you want to use a cutoff of $\alpha=0.001$? If so, then yes, $3.8 \times 10^{-9} \ll 0.001 $.
Irrational numbers are confusing for me
Not sure what you mean here. Mathematically, an "irrational" number is something like $\sqrt{2}$, a number that can't be represented as a ratio of whole numbers. Maybe you're confused by the 3.84e09 notation, where AeB stands for "A times 10 raised to the power of B" (?)
and I also have a negative t-value that is throwing me off.
A negative t-statistic means that the estimated parameter value is that many standard errors below zero. There's no difference between positive and negative t-statistics for standard two-tailed significance tests.
I'm also confused at the bottom where it shows: p-value: < 2.2e-16, what does this mean? Overall for the model?
Yes, that's a p-value for the full model vs. the null hypothesis of a constant model with $\hat y$ equal to the mean of the response variable.
