Should I include latent construct for total score in CFA?

Question

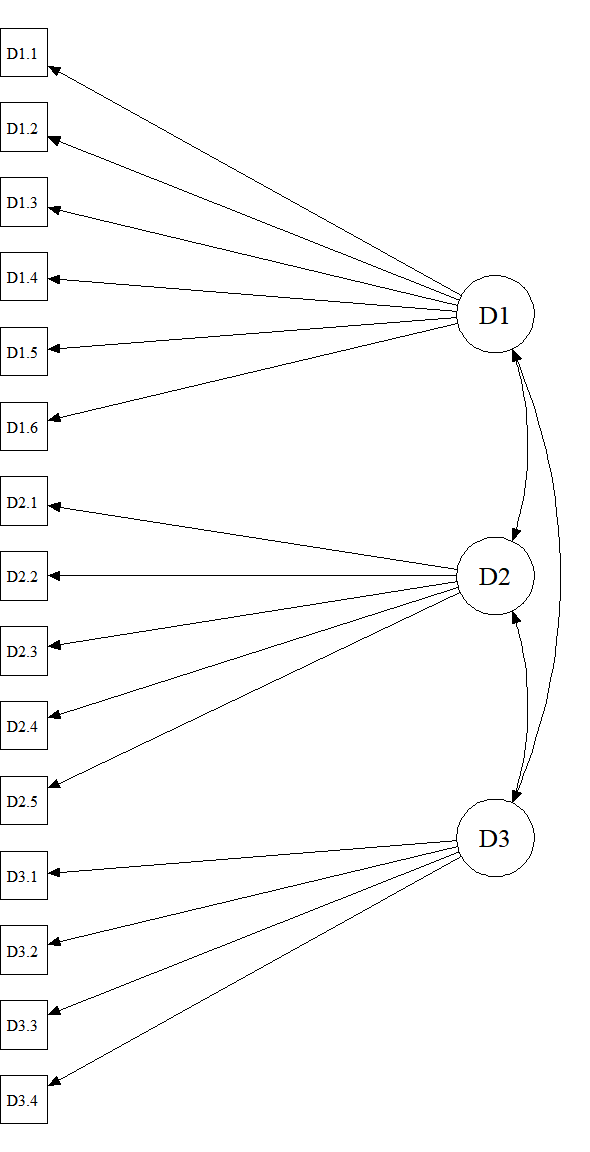
I need to validate factor structure for existing questionnaire that was translated to another language. Items of the questionnaire are organized into 3 subscales, say D1, D2 and D3. Total score, D, can also be computed (according to manual to original version of questionnaire) as a sum of 3 subscales.

So I run confirmatory factor analysis. My question is, shoud I include latent construct for total score (with arrows pointing from it to 3 subscales on path diagram) to CFA, like this:

My first reasoning was that I shouldn't because my primary question is if split into 3 subscales form original version is valid in new version. So this do not involve total score at all, like this:

Answer 1

It's not absolutely clear to me what you are proposing. I think the answer is no.

You should have three latent variables, representing the three subscales, and these should be correlated. The latents should be correlated. Each latent has arrows from it to it to the measured variables.

If you can post a path diagram, it would be clearer and I (or someone else) could help more.

Ah, thanks for the edit. No. You should not include the latent variable D AND the covariances between D1, D2 and D3. If you try to estimate that model, it will not be identified and will not converge.

One way to think abou this is that in your structural parameter you have three latent variables - this means you have three covariances to estimate, so you can only estimate these three parameters.

You can estimate the covariances directly (as in the second model) or you can remove these covariances and add a second order factor (D in the first path diagram). These two models are equivalent, and will give you the same model fits; in addition, all of the (first order) factor loadings will not change, and the the three parmeters (either loadings, or covariances) will be a transformation of each other.