Why is mean ± 2*SEM (95% confidence interval) overlapping, but the p-value is 0.05?

Question

I have data as two lists:

acol = [8.48, 9.82, 9.66, 9.81, 9.23, 10.35, 10.08, 11.05, 8.63, 9.52, 10.88, 10.05, 10.45, 10.0, 9.97, 12.02, 11.48, 9.53, 9.98, 10.69, 10.29, 9.74, 8.92, 11.94, 9.04, 11.42, 8.88, 10.62, 9.38, 12.56, 10.53, 9.4, 11.53, 8.23, 12.09, 9.37, 11.17, 11.33, 10.49, 8.32, 11.29, 10.31, 9.94, 10.27, 9.98, 10.05, 10.07, 10.03, 9.12, 11.56, 10.88, 10.3, 11.32, 8.09, 9.34, 10.46, 9.35, 11.82, 10.29, 9.81, 7.92, 7.84, 12.22, 10.42, 10.45, 9.33, 8.24, 8.69, 10.31, 11.29, 9.31, 9.93, 8.21, 10.32, 9.72, 8.95, 9.49, 8.11, 8.33, 10.41, 8.38, 10.31, 10.33, 8.83, 7.84, 8.11, 11.11, 9.41, 9.32, 9.42, 10.57, 9.74, 11.35, 9.44, 10.53, 10.08, 10.92, 9.72, 7.83, 11.09, 8.95, 10.69, 11.85, 10.19, 8.49, 9.93, 10.39, 11.08, 11.27, 8.71, 9.62, 11.75, 8.45, 8.09, 11.54, 9.0, 9.61, 10.82, 10.36, 9.22, 9.36, 10.38, 9.53, 9.2, 10.36, 9.38, 7.68, 9.99, 10.61, 8.81, 10.09, 10.24, 9.21, 10.17, 10.32, 10.41, 8.77]

bcol = [12.48, 9.76, 9.63, 10.86, 11.63, 9.07, 12.01, 9.52, 10.05, 8.66, 10.85, 9.87, 11.14, 10.59, 9.24, 9.85, 9.62, 11.54, 11.1, 9.38, 9.24, 9.68, 10.02, 9.91, 10.66, 9.7, 11.06, 9.27, 9.08, 11.31, 10.9, 10.63, 8.98, 9.81, 9.69, 10.71, 10.43, 10.89, 8.96, 9.74, 8.33, 11.45, 9.61, 9.59, 11.25, 9.44, 10.05, 11.63, 10.16, 11.71, 9.1, 9.53, 9.76, 9.33, 11.53, 11.59, 10.21, 10.68, 8.99, 9.44, 9.82, 10.35, 11.22, 9.05, 9.18, 9.57, 11.43, 9.4, 11.45, 8.39, 11.32, 11.16, 12.47, 11.62, 8.77, 11.34, 11.77, 9.53, 10.54, 8.73, 9.97, 9.98, 10.8, 9.6, 9.6, 9.96, 12.17, 10.01, 8.69, 8.94, 9.24, 9.84, 10.39, 10.65, 9.31, 9.93, 10.41, 8.5, 8.64, 10.23, 9.94, 10.47, 8.95, 10.8, 9.84, 10.26, 11.0, 11.22, 10.72, 9.14, 10.06, 11.52, 10.21, 9.82, 10.81, 10.3, 9.81, 11.48, 8.51, 9.55, 10.41, 12.17, 9.9, 9.07, 10.51, 10.26, 10.62, 10.84, 9.67, 9.75, 8.84, 9.85, 10.41, 9.18, 10.93, 11.41, 9.52]

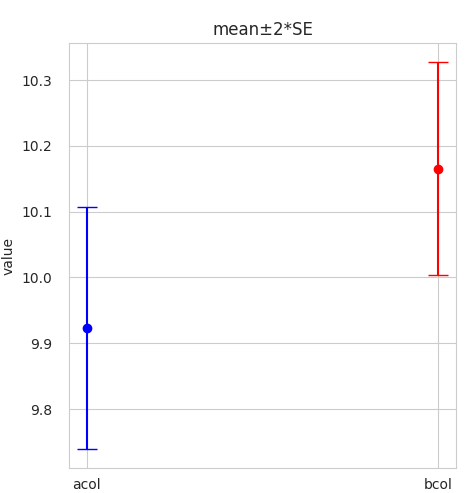
A summary of the above lists is given below:

N,   Mean, SD,   SEM,   95% CIs
137  9.92  1.08  0.092  (9.74, 10.1)
137  10.2  0.951 0.081  (10.0, 10.3)

An unpaired t-test for the above data gives a p-value of 0.05:

f,p = scipy.stats.ttest_ind(acol, bcol)
print(f, p)
-1.9644209241736 0.050499295018989004

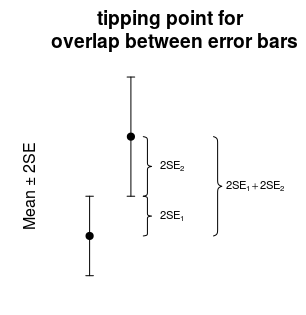
I understand from this and other pages that mean ± 2 * SEM (standard error of mean as calculated by SD/sqrt(N)) gives a 95% confidence interval (CI) range.

I also believe that if 95% confidence intervals are overlapping, P-value will be > 0.05.

I plotted the above data as mean ± 2 * SEM:

The 95% confidence intervals are overlapping. So why is the p-value reaching a significant level?

Answer 1

The overlap is just a (strict/inaccurate) rule of thumb

The point when the error bars do not overlap is when the distance between the two points is equal to $2(SE_1+SE_2)$. So effectively you are testing whether some sort of standardized score (distance divided by the sum of standard errors) is greater than 2. Let's call this $z_{overlap}$

$$ z_{overlap} = \frac{\vert \bar{X}_1- \bar{X}_2 \vert}{SE_1+SE_2} \geq 2$$

If this $z_{overlap} \geq 2$ then the error bars do not overlap.

---

The standard deviation of a linear sum of independent variables

Adding the standard deviations (errors) together is not the typical way to compute the standard deviation (error) of a linear sum (the parameter $\bar{X}_1-\bar{X}_2$ can be considered as a linear sum where one of the two is multiplied by a factor $-1$) See also: Sum of uncorrelated variables

So the following are true for independent $\bar{X}_1$ and $\bar{X}_2$:

$$\begin{array}{} \text{Var}(\bar{X}_1-\bar{X}_2) &=& \text{Var}(\bar{X}_1) + \text{Var}(\bar{X}_2)\\ \sigma_{\bar{X}_1-\bar{X}_2}^2 &=& \sigma_{\bar{X}_1}^2+\sigma_{\bar{X}_2}^2\\ \sigma_{\bar{X}_1-\bar{X}_2} &=& \sqrt{\sigma_{\bar{X}_1}^2+\sigma_{\bar{X}_2}^2}\\ \text{S.E.}(\bar{X}_1-\bar{X}_2) &=& \sqrt{\text{S.E.}(\bar{X}_1)^2 + \text{S.E.}(\bar{X}_2)^2}\\ \end{array}$$

But not

$$\text{S.E.}(\bar{X}_1-\bar{X}_2) \neq {\text{S.E.}(\bar{X}_1) + \text{S.E.}(\bar{X}_2)}$$

---

'Correct' formula for comparing the difference in the mean of two samples

For a t-test to compare the difference in means of two populations, you should be using a formula like

• In the simplest case: $$t = \frac{\bar{X}_1 - \bar{X}_2}{\sqrt{SE_1^2+SE_2^2}}$$ this is when we consider the variances to be unequal or when the sample sizes are equal.

• If the sample sizes are different and you consider the variance of the populations to be equal, then you can estimate the variances for both samples together instead of separately, and use one of many formulae for the pooled variance like

  $$s_p = \sqrt{\frac{(n_1-1)s_1^2 +(n_2-1)s_2^2}{n_1+n_2-2}}$$

  with $$t = \frac{\bar{X}_1 - \bar{X}_2}{s_p \sqrt{\frac{1}{n_1}+\frac{1}{n_2}}}$$

  and with $SE_1 = s_1/\sqrt{n_1}$ and $SE_2 = s_2/\sqrt{n_2}$ you get

  $$t = \frac{\bar{X}_1 - \bar{X}_2}{\sqrt{\frac{n_1+n_2}{n_1+n_2-2} \left( \frac{n_1-1}{n_2} SE_1^2 + \frac{n_2-1}{n_1} SE_2^2 \right)}}$$

Note that the value $\sqrt{SE_1^2+SE_2^2}$ is smaller than $SE_1+SE_2$, therefore $t>z_{overlap}$.

Sidenotes:

• In the case of the pooled variance, you might have a situation - although it is rare - that the variance of the larger sample is larger than the variance of the smaller sample, and then it is possible that $t<z_{overlap}$.

• Instead of z-values and a z-test you are actually doing (should be doing) a t-test. So it might be that the levels on which you base the confidence intervals for the error bars (like '95% is equivalent to 2 times the standard error') will be different for the t-test. To be fair, to compare apples with apples, you should use the same standard and base the confidence levels for the error bars on a t-test as well. So let's assume that also for the t-test the boundary level that relates to 95% is equal to or less than 2 (this is the case for sample sizes larger than 60).

If this $t \geq 2$ then the difference is significant (at a 5% level).

The standard error of the difference between two variables is not the sum of standard errors of each variable. This sum is overestimating the error for the difference and will be too conservative (too often claim there is no significant difference).

So $t>z_{overlap}$ and may lead to a significant difference while the error bars have overlap. You do not need non-overlapping error bars in order to have a significant difference. This overlap is a stricter requirement and happens when the p-value is $\leq 0.05$ (and it will often be a lower p-value).

Answer 2

The p-value should be considered between a CI and a parameter value, not two CIs. Indeed, the red point falls entirely outside the blue CI, and the blue point falls entirely outside the red CI.

And it is true that under the null hypothesis such an event would happen 5% of the time:

• 2.5% of the time, you get a point above the 95% CI
• 2.5% of the time, you get a point below the 95% CI

If it is only the whiskers that overlap or touch, then the null hypothesis will produce this result a lot less often than 5%. This is because (to use your example) both the blue sample would need to be low, and at the same time the red sample would need to be high (exactly how high would depend on the blue value). You can picture it as a 3D multivariate Gaussian plot, with no skew since the two errors are independent of one another:

Along each axis the probability of falling outside the highlighted region (the CI) is 0.05. But the total probabilities of the blue and pink areas, which gives you P of the two CIs barely touching, is less than 0.05 in your case.

A change of variables from the blue/red axes to the green one will let you integrate this volume using a univariate rather than multivariate Gaussian, and the new variance is the pooled variance from @Sextus-Empiricus's answer.

Answer 3

Even if we ignore the difference between confidence and probability, the overlap consists of points for which both the red probability and the blue probability are greater than 0.05. But that doesn't mean that the probability of both is greater than 0.05. For instance, if both the red and blue probability are 0.10, then the joint probability (assuming independence) is 0.01. If you integrate over the whole overlap, this will be less than 0.01.

When you look at the overlap, you are seeing points for which the difference is less than two standard deviations. But remember that the variance of the difference between two variables is the sum of the individual variances. So you can generally use a rule of thumb that if you want to compare two different populations by checking for overlapping CI, you need to divide the size of each CI by $\sqrt 2$: if the variances are of similar sizes, then the variance of the difference will be twice the individual variances, and the standard deviation will be $\sqrt 2$ times as large.