How should you make a box plot when the data have an outlier? Must we use the data with the outlier, or use the data without the outlier? If we use the data without the outlier, we will change the quartiles, won't we?
$\begingroup$
$\endgroup$
2
-
2$\begingroup$ How much data do you have? 1 outlier is unlikely to have a material effect on your boxplot. $\endgroup$– gung - Reinstate MonicaCommented May 19, 2015 at 16:14
-
3$\begingroup$ It is difficult to understand what you are getting at here. Box plot functions (commands, routines, whatever) have their own ideas on what is an outlier and show such points individually. Some of the value of a box plot is that it flags points that may be outliers. What you then do is a set of forking paths and we can't tell you in advance which to take. $\endgroup$– Nick CoxCommented May 19, 2015 at 16:23
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
If you're talking about something like this:
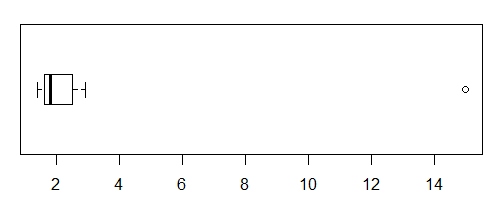
$\qquad^{\text{[Data are } \tt{1.4, 1.5, 1.7, 1.8, 2.1,2.9, 15 } \text{ ]}}$
then there's probably no real need to remove anything; the display adequately indicates what it's designed to show. If the comparisons you want to make can be made there's not really a problem to solve.
However, sometimes a really extreme value can almost completely obscure the rest of the information:
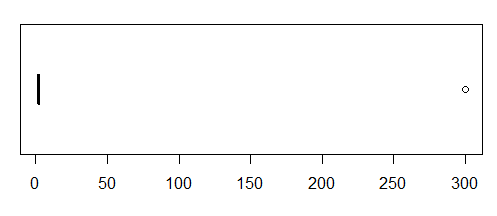
$\qquad^{\text{[Data are } \tt{1.4, 1.5, 1.7, 1.8, 2.1,2.9, 300 } \text{ ]}}$
A useful comparison between two sets of numbers which apart from the outlier all lie in a small range (but for which clear differences exist, even though they can't be seen on a plot like that) would be lost.
And in that case you might consider any of a number of alternatives - omitting the extreme value but explicitly mentioning it in the figure text, transformation, including a full scale break, or including some indication on the plot of another point outside the range of the figure, such as an arrow (and a mention on the plot of the omitted value or values).
These alternatives are discussed more fully here
So in short: only do something like that if it's really necessary to do so ... but whatever you do, the omission of any values must be quite clear to anyone looking at the figure.
